6.3 Reduction formula | Trigonometry

6.3 Reduction formula (EMBHJ)

Any trigonometric function whose argument is $\text{90}\text{°}±\theta$ ; $\text{180}\text{°}±\theta$ and $\text{360}\text{°}±\theta$ can be written simply in terms of $\theta$ .

Deriving reduction formulae (EMBHK)

Reduction formulae for function values of $\text{180}\text{°}±\theta$

Function values of $\text{180}\text{°}-\theta$

In the figure $P(\sqrt{3};1)$ and $P'$ lie on the circle with radius $\text{2}$ . $OP$ makes an angle $\theta =\text{30}\text{°}$ with the $x$ -axis.
1. If points $P$ and $P'$ are symmetrical about the $y$ -axis, determine the coordinates of $P'$ .
2. Write down values for $\sin \theta$ , $\cos \theta$ and $\tan \theta$ .
3. Use the coordinates for $P'$ to determine $\sin(\text{180}\text{°}-θ)$ , $\cos(\text{180}\text{°}-θ)$ , $\tan(\text{180}\text{°}-θ)$ .
4. From your results determine a relationship between the trigonometric function values of $\left(\text{180}\text{°}-\theta \right)$ and $\theta$ .
Function values of $\text{180}\text{°}+\theta$

In the figure $P(\sqrt{3};1)$ and $P'$ lie on the circle with radius $\text{2}$ . $OP$ makes an angle $\theta =\text{30}\text{°}$ with the $x$ -axis.
1. If points $P$ and $P'$ are symmetrical about the origin (the two points are symmetrical about both the $x$ -axis and the $y$ -axis), determine the coordinates of $P'$ .
2. Use the coordinates for $P'$ to determine $\sin (\text{180}\text{°}+θ)$ , $\cos (\text{180}\text{°}+θ)$ and $\tan (\text{180}\text{°}+θ)$ .
3. From your results determine a relationship between the trigonometric function values of $\left(\text{180}\text{°}+\theta \right)$ and $\theta$ .
Complete the following reduction formulae:
1. $\sin (\text{180}\text{°} - \theta) = \ldots \ldots$
2. $\cos (\text{180}\text{°} - \theta) = \ldots \ldots$
3. $\tan (\text{180}\text{°} - \theta) = \ldots \ldots$
4. $\sin (\text{180}\text{°} + \theta) = \ldots \ldots$
5. $\cos (\text{180}\text{°} + \theta) = \ldots \ldots$
6. $\tan (\text{180}\text{°} + \theta) = \ldots \ldots$

Worked example 7: Reduction formulae for function values of $\text{180}\text{°} \pm \theta$

Write the following as a single trigonometric ratio:

$\frac{\sin \text{163}\text{°}}{\cos \text{197}\text{°}} + \tan \text{17}\text{°} + \cos (\text{180}\text{°} - \theta) \times \tan (\text{180}\text{°} + \theta)$

Use reduction formulae to write the trigonometric function values in terms of acute angles and $\theta$

$= \frac{\sin (\text{180}\text{°} - \text{17}\text{°})}{\cos (\text{180}\text{°} + \text{17}\text{°})} + \tan \text{17}\text{°} + (- \cos \theta) \times \tan \theta$

Simplify

$\begin{align*} &= \frac{\sin \text{17}\text{°}}{- \cos \text{17}\text{°}} + \tan \text{17}\text{°} - \cos \theta \times \frac{\sin \theta}{\cos \theta} \\ &= - \tan \text{17}\text{°} + \tan \text{17}\text{°} - \sin \theta \\ &= -\sin \theta \end{align*}$

Reduction formulae for function values of $\text{180}\text{°} \pm \theta$

Textbook Exercise 6.3

$\tan \text{150}\text{°}\sin \text{30}\text{°} - \cos \text{210}\text{°}$

\begin{align*} &\tan \text{150}\text{°}\sin \text{30}\text{°} - \cos \text{210}\text{°} \\ &= \tan (\text{180}\text{°} - \text{30}\text{°})\sin \text{30}\text{°} - \cos (\text{180}\text{°} + \text{30}\text{°}) \\ &= - \tan \text{30}\text{°} \sin \text{30}\text{°} + \cos \text{30}\text{°} \\ &= - \left( \frac{1}{\sqrt{3}} \right) \left( \frac{1}{2} \right) + \frac{\sqrt{3}}{2} \\ &= - \frac{1}{2\sqrt{3}} + \frac{\sqrt{3}}{2} \\ &= - \frac{1}{2\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} + \frac{\sqrt{3}}{2} \\ &= - \frac{\sqrt{3}}{6} + \frac{\sqrt{3}}{2} \\ &= \frac{-\sqrt{3} + 3\sqrt{3}}{6} \\ &= \frac{2\sqrt{3}}{6} \\ &= \frac{\sqrt{3}}{3} \end{align*}

$(1 + \cos \text{120}\text{°})(1 - \sin^2 \text{240}\text{°})$

\begin{align*} &(1 + \cos \text{120}\text{°})(1 - \sin^2 \text{240}\text{°}) \\ &= \left( 1 + \cos (\text{180}\text{°} - \text{60}\text{°}) \right) \left( 1 - \sin^2 (\text{180}\text{°} + \text{60}\text{°}) \right) \\ &= \left( 1 - \cos \text{60}\text{°} \right) \left( 1 - \sin^2 \text{60}\text{°} \right) \\ &= \left( 1 - \frac{1}{2} \right) \left( 1 - \left( \frac{\sqrt{3}}{2} \right)^2 \right) \\ &= \left( \frac{1}{2} \right) \left( 1 - \frac{3}{4} \right) \\ &= \frac{1}{2} \times \frac{1}{4} \\ &= \frac{1}{8} \end{align*}

$\cos^2 \text{140}\text{°} + \sin^2 \text{220}\text{°}$

\begin{align*} &\cos^2 \text{140}\text{°} + \sin^2 \text{220}\text{°} \\ &= \cos^2 (\text{180}\text{°} - \text{40}\text{°})+ \sin^2 (\text{180}\text{°} + \text{40}\text{°}) \\ &= \cos^2 \text{40}\text{°} + \sin^2 \text{40}\text{°} \\ &= \text{1} \end{align*}

$\tan (\text{180}\text{°} - \theta) \times \sin (\text{180}\text{°} + \theta)$

\begin{align*} &\tan (\text{180}\text{°} - \theta) \times \sin (\text{180}\text{°} + \theta) \\ &= - \tan \theta \times \left( - \sin \theta \right) \\ &= - \frac{\sin \theta}{\cos \theta} \times \left( - \sin \theta \right) \\ &= \frac{\sin^2 \theta}{\cos \theta} \\ &= \frac{1 - \cos^2 \theta}{\cos \theta} \end{align*}

$\dfrac{\tan (\text{180}\text{°} + \theta) \cos (\text{180}\text{°} - \theta)}{\sin (\text{180}\text{°} - \theta)}$

\begin{align*} \dfrac{\tan (\text{180}\text{°} + \theta) \cos (\text{180}\text{°} - \theta)}{\sin (\text{180}\text{°} - \theta)} &= -\dfrac{ \tan \theta \cos \theta }{\sin \theta } \\ &= -\dfrac{ \frac{\sin \theta}{\cos \theta} \cos \theta }{\sin \theta } \\ &= -\dfrac{ \sin \theta }{\sin \theta } \\ &= -\text{1} \end{align*}

$\tan \text{140}\text{°} + 3 \tan \text{220}\text{°}$

\begin{align*} &\tan \text{140}\text{°} + 3 \tan \text{220}\text{°} \\ &= \tan (\text{180}\text{°} - \text{40}\text{°}) + 3 \tan (\text{180}\text{°} + \text{40}\text{°}) \\ &= - \tan \text{40}\text{°} + 3 \tan \text{40}\text{°} \\ &= 2 \tan \text{40}\text{°} \\ &= 2t \end{align*}

$\frac{\cos \text{220}\text{°}}{\sin \text{140}\text{°}}$

\begin{align*} \dfrac{\cos \text{220}\text{°}}{\sin \text{140}\text{°}} &= \dfrac{\cos (\text{180}\text{°} + \text{40}\text{°})}{\sin (\text{180}\text{°} - \text{40}\text{°})} \\ &= \dfrac{ - \cos \text{40}\text{°} }{\sin \text{40}\text{°} } \\ &= - \left( \dfrac{ \sin \text{40}\text{°} }{\cos \text{40}\text{°} } \right)^{-1} \\ &= - \left( \tan \text{40}\text{°} \right)^{-1} \\ &= - \dfrac{ 1 }{\tan \text{40}\text{°} } \\ &= - \frac{ 1 }{t} \end{align*}

Reduction formulae for function values of ( $\text{360}\text{°} \pm \theta$ ) and $(- \theta)$

Function values of ( $\text{360}\text{°} - \theta$ ) and $(- \theta)$

In the Cartesian plane we measure angles from the positive $x$ -axis to the terminal arm, which means that an anti-clockwise rotation gives a positive angle. We can therefore measure negative angles by rotating in a clockwise direction.

For an acute angle $\theta$ , we know that $-\theta$ will lie in the fourth quadrant.

In the figure $P(\sqrt{3};1)$ and $P'$ lie on the circle with radius $\text{2}$ . $OP$ makes an angle $\theta =\text{30}\text{°}$ with the $x$ -axis.
1. If points $P$ and $P'$ are symmetrical about the $x$ -axis ( $y = 0$ ), determine the coordinates of $P'$ .
2. Use the coordinates of $P'$ to determine $\sin(\text{360}\text{°}-θ)$ , $\cos(\text{360}\text{°}-θ)$ and $\tan(\text{360}\text{°}-θ)$ .
3. Use the coordinates of $P'$ to determine $\sin (-\theta)$ , $\cos (-\theta)$ and $\tan (-\theta)$ .
4. From your results determine a relationship between the function values of $\left(\text{360}\text{°}-\theta \right)$ and $- \theta$ .
5. Complete the following reduction formulae:
  1. $\sin (\text{360}\text{°} - \theta) = \ldots \ldots$
  2. $\cos (\text{360}\text{°} - \theta) = \ldots \ldots$
  3. $\tan (\text{360}\text{°} - \theta) = \ldots \ldots$
  4. $\sin (- \theta) = \ldots \ldots$
  5. $\cos (- \theta) = \ldots \ldots$
  6. $\tan (- \theta) = \ldots \ldots$
Function values of $\text{360}\text{°} + \theta$

We can also have an angle that is larger than $\text{360}\text{°}$ . The angle completes a revolution of $\text{360}\text{°}$ and then continues to give an angle of $\theta$ .

Complete the following reduction formulae:
1. $\sin (\text{360}\text{°} + \theta) = \ldots \ldots$
2. $\cos (\text{360}\text{°} + \theta) = \ldots \ldots$
3. $\tan (\text{360}\text{°} + \theta) = \ldots \ldots$

From working with functions, we know that the graph of $y = \sin \theta$ has a period of $\text{360}\text{°}$ . Therefore, one complete wave of a sine graph is the same as one complete revolution for $\sin \theta$ in the Cartesian plane.

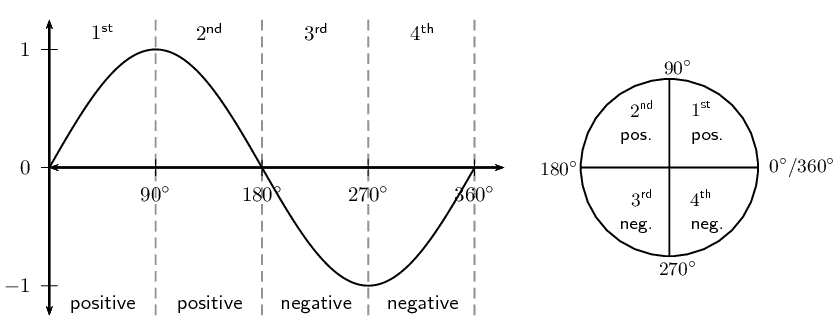
We can also have multiple revolutions. The periodicity of the trigonometric graphs shows this clearly. A complete sine or cosine curve is completed in $\text{360}\text{°}$ .

If $k$ is any integer, then

$\begin{align*} \sin (k \cdot \text{360}\text{°} + \theta) &= \sin \theta \\ \cos (k \cdot \text{360}\text{°} + \theta) &= \cos \theta \\ \tan (k \cdot \text{360}\text{°} + \theta) &= \tan \theta \end{align*}$

Worked example 8: Reduction formulae for function values of $\text{360}\text{°} \pm \theta$

If $f = \tan \text{67}\text{°}$ , express the following in terms of $f$

$\frac{\sin \text{293}\text{°}}{\cos \text{427}\text{°}} + \tan(-\text{67}\text{°}) + \tan \text{1 147}\text{°}$

Using reduction formula

$\begin{align*} \phantom{=} \frac{\sin \text{293}\text{°}}{\cos \text{427}\text{°}} + \tan(-\text{67}\text{°}) + \tan \text{1 147}\text{°} &= \frac{\sin (\text{360}\text{°} - \text{67}\text{°})}{\cos (\text{360}\text{°} + \text{67}\text{°})} - \tan(\text{67}\text{°}) + \tan \left( 3(\text{360}\text{°}) + \text{67}\text{°} \right) \\ &= \frac{- \sin \text{67}\text{°}}{\cos \text{67}\text{°}} - \tan \text{67}\text{°} + \tan \text{67}\text{°} \\ &= - \tan \text{67}\text{°} \\ &= - f \end{align*}$

Worked example 9: Using reduction formula

Evaluate without using a calculator:

$\tan^2 \text{210}\text{°} - (1 + \cos \text{120}\text{°}) \sin^2 \text{405}\text{°}$

Simplify the expression using reduction formulae and special angles

$\begin{align*} \tan^2 \text{210}\text{°} - (1 + \cos \text{120}\text{°}) \sin^2 \text{405}\text{°} &= \tan^2 (\text{180}\text{°} + \text{30}\text{°}) - \left( 1 + \cos (\text{180}\text{°} - \text{60}\text{°}) \right) \sin^2 (\text{360}\text{°} + \text{45}\text{°}) \\ &= \tan^2 \text{30}\text{°} - \left(1 + ( - \cos\text{60}\text{°}) \right) \sin^2 \text{45}\text{°} \\ &= \left( \frac{1}{\sqrt{3}} \right)^2 - \left(1 - \frac{1}{2} \right) \left( \frac{1}{\sqrt{2}} \right)^2 \\ &= \frac{1}{3} - \left(\frac{1}{2} \right) \left( \frac{1}{2} \right) \\ &= \frac{1}{3} - \frac{1}{4} \\ &= \frac{1}{12} \end{align*}$

Using reduction formula

Textbook Exercise 6.4

$\dfrac{ \tan (\text{180}\text{°} - \theta) \sin (\text{360}\text{°} + \theta)}{\cos (\text{180}\text{°} + \theta) \tan (\text{360}\text{°} - \theta)}$

\begin{align*} &\dfrac{ \tan (\text{180}\text{°} - \theta) \sin (\text{360}\text{°} + \theta)}{\cos (\text{180}\text{°} + \theta) \tan (\text{360}\text{°} - \theta)} \\ &= \dfrac{ -\tan \theta \sin \theta }{(-\cos \theta )(- \tan \theta )} \\ &=-\dfrac{ \sin \theta }{ \cos \theta } \\ &= - \tan \theta \end{align*}

$\cos^2 (\text{360}\text{°} + \theta) + \cos (\text{180}\text{°} + \theta) \tan (\text{360}\text{°} - \theta) \sin (\text{360}\text{°} + \theta)$

\begin{align*} &\cos^2 (\text{360}\text{°} + \theta) + \cos (\text{180}\text{°} + \theta) \tan (\text{360}\text{°} - \theta) \sin (\text{360}\text{°} + \theta) \\ &= \cos^2 \theta + (- \cos \theta )(- \tan \theta) (\sin \theta ) \\ &= \cos^2 \theta + ( \cos \theta )( \frac{\sin \theta }{\cos \theta }) (\sin \theta ) \\ &= \cos^2 \theta + \sin^2 \theta \\ &= \text{1} \end{align*}

$\dfrac{\sin (\text{360}\text{°} + \alpha) \tan (\text{180}\text{°} + \alpha)}{\cos (\text{360}\text{°} - \alpha) \tan^2 (\text{360}\text{°} + \alpha)}$

\begin{align*} &\dfrac{\sin (\text{360}\text{°} + \alpha) \tan (\text{180}\text{°} + \alpha)}{\cos (\text{360}\text{°} - \alpha) \tan^2 (\text{360}\text{°} + \alpha)} \\ &= \dfrac{\sin \alpha \tan \alpha }{\cos \alpha \tan^2 \alpha }\\ &= \dfrac{\sin \alpha \frac{\sin \alpha}{\cos \alpha} }{\cos \alpha \frac{\sin^2 \alpha}{\cos^2 \alpha} }\\ &= \dfrac{\frac{\sin^2 \alpha}{\cos \alpha} }{ \frac{\sin^2 \alpha}{\cos \alpha} }\\ &= \dfrac{\sin^2 \alpha}{\cos \alpha} \times \frac{\cos \alpha}{\sin^2 \alpha} \\ &= \text{1} \end{align*}

Write the following in terms of $\cos \beta$:

$\dfrac{\cos (\text{360}\text{°} - \beta) \cos (-\beta) - 1}{\sin (\text{360}\text{°} + \beta) \tan (\text{360}\text{°} - \beta)}$

\begin{align*} &\dfrac{\cos (\text{360}\text{°} - \beta) \cos (-\beta) - 1}{\sin (\text{360}\text{°} + \beta) \tan (\text{360}\text{°} - \beta)} \\ &= \dfrac{\cos\beta \cos\beta-1}{(-\sin\beta)( -\tan\beta)} \\ &= \dfrac{-(1-\cos^2\beta)}{\sin\beta \frac{\sin\beta}{\cos\beta}} \\ &=\dfrac{-\sin^2\beta}{\sin^2\beta}\times\frac{\cos\beta}{1} \\ &=-\cos\beta \end{align*}

$\dfrac{\cos \text{300}\text{°} \tan \text{150}\text{°} }{\sin \text{225}\text{°} \cos (-\text{45}\text{°})}$

\begin{align*} &\dfrac{\cos \text{300}\text{°} \tan \text{150}\text{°} }{\sin \text{225}\text{°} \cos (-\text{45}\text{°})} \\ &= \dfrac{\cos (\text{360}\text{°}-\text{60}\text{°}) \tan (\text{180}\text{°}-\text{30}\text{°}) }{\sin (\text{180}\text{°}+\text{45}\text{°}) \cos (-\text{45}\text{°})} \\ &= \dfrac{\cos \text{60}\text{°}(- \tan \text{30}\text{°}) }{(-\sin \text{45}\text{°}) \cos \text{45}\text{°} } \\ &= \dfrac{\frac{1}{2}(\frac{1}{\sqrt{3}}) }{\frac{1}{\sqrt{2}} \left( \frac{1}{\sqrt{2}} \right) } \\ &= \dfrac{\frac{1}{2}(\frac{1}{\sqrt{3}}) }{\frac{1}{2} } \\ &= \frac{1}{\sqrt{3}} \end{align*}

$3 \tan \text{405}\text{°} + 2 \tan \text{330}\text{°} \cos \text{750}\text{°}$

\begin{align*} &3 \tan \text{405}\text{°} + 2 \tan \text{330}\text{°} \cos \text{750}\text{°} \\ &=3\tan(\text{360}\text{°}+\text{45}\text{°})+2\tan(\text{360}\text{°}-\text{30}\text{°})\cos \left( 2(\text{360}\text{°})+\text{30}\text{°} \right) \\ &=3\tan\text{45}\text{°}+2(-\tan\text{30}\text{°}) \cos\text{30}\text{°} \\ &=3\tan\text{45}\text{°}-2(\frac{\sin\text{30}\text{°}}{\cos\text{30}\text{°}}) \cos\text{30}\text{°} \\ &=3\tan\text{45}\text{°}-2\sin\text{30}\text{°} \\ &=3(1) - 2 \left( \frac{1}{2} \right) \\ &=\text{2} \end{align*}

$\dfrac{\cos \text{315}\text{°} \cos \text{405}\text{°} + \sin \text{45}\text{°} \sin \text{135}\text{°}}{\sin \text{750}\text{°}}$

\begin{align*} &\dfrac{\cos \text{315}\text{°} \cos \text{405}\text{°} + \sin \text{45}\text{°} \sin \text{135}\text{°}}{\sin \text{750}\text{°}} \\ &=\dfrac{\cos(\text{360}\text{°}-\text{45}\text{°}) \cos (\text{360}\text{°}+\text{45}\text{°}) + \sin \text{45}\text{°} \sin (\text{180}\text{°}-\text{45}\text{°}) }{\sin \left( 2(\text{360}\text{°})+\text{30}\text{°} \right) }\\ &=\dfrac{\cos\text{45}\text{°} \cos\text{45}\text{°} + \sin \text{45}\text{°} \sin -\text{45}\text{°} }{\sin \text{30}\text{°} }\\ &=\dfrac{\cos^2 \text{45}\text{°}+ \sin^2 \text{45}\text{°}}{\sin \text{30}\text{°} }\\ &=\dfrac{1}{\frac{1}{2} }\\ &=\text{2} \end{align*}

$\tan\text{150}\text{°} \cos \text{390}\text{°} - 2 \sin \text{510}\text{°}$

\begin{align*} &\tan\text{150}\text{°} \cos \text{390}\text{°} - 2 \sin \text{510}\text{°} \\ &= \tan(\text{180}\text{°}-\text{30}\text{°}) \cos (\text{360}\text{°}+\text{30}\text{°}) - 2 \sin \left( 2(\text{360}\text{°})+\text{150}\text{°} \right)\\ &= -\tan \text{30}\text{°} \cos \text{30}\text{°} - 2 \sin \text{150}\text{°} \\ &= -\frac{\sin \text{30}\text{°}}{\cos \text{30}\text{°}} \cos \text{30}\text{°} - 2 \sin (\text{180}\text{°}-\text{30}\text{°}) \\ &= -\sin \text{30}\text{°} - 2 \sin\text{30}\text{°} \\ &= -3 \left( \frac{1}{2} \right) \\ &=-\frac{3}{2} \end{align*}

$\dfrac{2 \sin \text{120}\text{°} + 3 \cos \text{765}\text{°} - 2 \sin \text{240}\text{°} - 3 \cos \text{45}\text{°}}{5 \sin \text{300}\text{°} + 3 \tan \text{225}\text{°} - 6 \cos \text{60}\text{°}}$

\begin{align*} &\dfrac{2 \sin \text{120}\text{°} + 3 \cos \text{765}\text{°} - 2 \sin \text{240}\text{°} - 3 \cos \text{45}\text{°}}{5 \sin \text{330}\text{°} + 3 \tan \text{225}\text{°} - 6 \cos \text{60}\text{°}} \\ &= \dfrac{2 \sin (\text{180}\text{°}-\text{60}\text{°})+3 \cos (2(\text{360}\text{°})+\text{45}\text{°})-2 \sin (\text{180}\text{°}+\text{60}\text{°})-3 \cos \text{45}\text{°}}{5 \sin (\text{360}\text{°}-\text{30}\text{°}) + 3 \tan (\text{180}\text{°}+\text{45}\text{°}) - 6 \cos \text{60}\text{°}}\\ &= \dfrac{2 \sin \text{60}\text{°}+3 \cos\text{45}\text{°}+2 \sin \text{60}\text{°} -3 \cos \text{45}\text{°}}{-5 \sin \text{30}\text{°} + 3 \tan \text{45}\text{°} - 6 \cos \text{60}\text{°}}\\ &= \dfrac{4 \sin \text{60}\text{°} }{-5 \sin \text{30}\text{°} + 3 \tan \text{45}\text{°} - 6 \cos \text{60}\text{°}}\\ &= \dfrac{4\left(\frac{\sqrt{3}}{2}\right) }{-5\left(\frac{1}{2}\right) + 3\left(1\right) - 6\left(\frac{1}{2}\right)}\\ &= \dfrac{2\sqrt{3}}{- \frac{5}{2} }\\ &= - 2\sqrt{3} \times \frac{2}{5} \\ &=-\frac{4\sqrt{3}}{5} \end{align*}

$\sin (-\alpha) = - \sin \alpha$

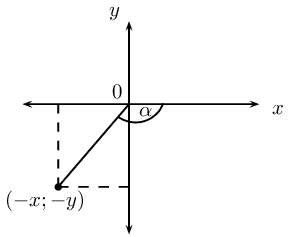
$\cos (-\alpha) = -\cos \alpha$

\begin{align*} \cos(-\alpha)&=\frac{-x}{r} \qquad \text{(lies in III quad)}\\ &=-\cos\alpha \end{align*}

$\sin \text{317}\text{°}$

\begin{align*} \sin \text{317}\text{°}&=\sin(\text{360}\text{°}-\text{43}\text{°}) \\ &=-\sin\text{43}\text{°} \\ &=-t \end{align*}

$\cos^2 \text{403}\text{°}$

\begin{align*} \cos^2 \text{403}\text{°}&=\cos^2(\text{360}\text{°}+\text{43}\text{°}) \\ &=\cos^2 \text{43}\text{°} \\ &=1-\sin^2 \text{43}\text{°} \\ &= 1-t^2 \end{align*}

$\tan (-\text{43}\text{°})$

\begin{align*} \tan (-\text{43}\text{°}) &= -\tan\text{43}\text{°} \\ &=-\frac{\sin\text{43}\text{°}}{\cos\text{43}\text{°}} \\ &=\pm\frac{t}{\sqrt{1-t^2}} \end{align*}

Reduction formulae for function values of $\text{90}\text{°}±\theta$

In any right-angled triangle, the two acute angles are complements of each other, $\hat{A} + \hat{C} = \text{90}\text{°}$

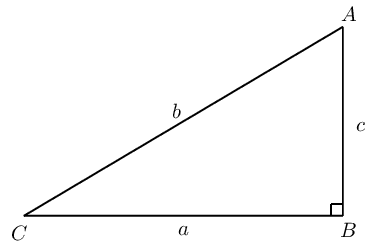
Complete the following:

In $\triangle ABC$

$\begin{align*} \sin \hat{C} &= \dfrac{c}{b} = \cos \ldots \\ \cos \hat{C} &= \dfrac{a}{b} = \sin \ldots \end{align*}$

Complementary angles are positive acute angles that add up to $\text{90}\text{°}$ . For example $\text{20}\text{°}$ and $\text{70}\text{°}$ are complementary angles.

In the figure $P(\sqrt{3};1)$ and $P'$ lie on a circle with radius $\text{2}$ . $OP$ makes an angle of $\theta =\text{30}\text{°}$ with the $x$ -axis.

Function values of $\text{90}\text{°}-\theta$
1. If points $P$ and $P'$ are symmetrical about the line $y = x$ , determine the coordinates of $P'$ .
2. Use the coordinates for $P'$ to determine $\sin (\text{90}\text{°} - \theta)$ and $\cos(\text{90}\text{°} - \theta)$ .
3. From your results determine a relationship between the function values of $\left(\text{90}\text{°}-\theta \right)$ and $\theta$ .
Function values of $\text{90}\text{°}+\theta$

In the figure $P(\sqrt{3};1)$ and $P'$ lie on the circle with radius $\text{2}$ . $OP$ makes an angle $\theta =\text{30}\text{°}$ with the $x$ -axis.
1. If point $P$ is rotated through $\text{90}\text{°}$ to get point $P'$ , determine the coordinates of $P'$ .
2. Use the coordinates for $P'$ to determine $\sin (\text{90}\text{°} + \theta)$ and $\cos(\text{90}\text{°} + \theta)$ .
3. From your results determine a relationship between the function values of $\left(\text{90}\text{°}+\theta \right)$ and $\theta$ .
Complete the following reduction formulae:
1. $\sin (\text{90}\text{°} - \theta) = \ldots \ldots$
2. $\cos (\text{90}\text{°} - \theta) = \ldots \ldots$
3. $\sin (\text{90}\text{°} + \theta) = \ldots \ldots$
4. $\cos (\text{90}\text{°} + \theta) = \ldots \ldots$

Sine and cosine are known as co-functions. Two functions are called co-functions if $f\left(A\right)=g\left(B\right)$ whenever $A+B=\text{90}\text{°}$ (that is, $A$ and $B$ are complementary angles).

The function value of an angle is equal to the co-function of its complement.

Thus for sine and cosine we have

$\begin{align*} \sin(\text{90}\text{°} - θ) &= \cos θ \\ \cos(\text{90}\text{°} - θ) &= \sin θ \end{align*}$

The sine and cosine graphs illustrate this clearly: the two graphs are identical except that they have a $\text{90}\text{°}$ phase difference.

Worked example 10: Using the co-function rule

Write each of the following in terms of $\sin \text{40}\text{°}$ :

$\cos \text{50}\text{°}$
$\sin \text{320}\text{°}$
$\cos \text{230}\text{°}$
$\cos \text{130}\text{°}$

$\cos \text{50}\text{°} = \sin ( \text{90}\text{°} - \text{50}\text{°}) = \sin \text{40}\text{°}$
$\sin \text{320}\text{°} = \sin (\text{360}\text{°} - \text{40}\text{°}) = -\sin \text{40}\text{°}$
$\cos \text{230}\text{°} = \cos (\text{180}\text{°} + \text{50}\text{°}) = -\cos \text{50}\text{°} = -\cos (\text{90}\text{°} - \text{40}\text{°}) = -\sin \text{40}\text{°}$
$\cos \text{130}\text{°} = \cos ( \text{90}\text{°} + \text{40}\text{°}) = -\sin \text{40}\text{°}$

Function values of $\theta -\text{90}\text{°}$

We can write $\sin (\theta - \text{90}\text{°})$ as

$\begin{align*} \sin (\theta - \text{90}\text{°}) &= \sin \left[-(\text{90}\text{°} - \theta)\right] \\ &= -\sin (\text{90}\text{°} - \theta) \\ &= -\cos\theta \end{align*}$

similarly, we can show that $\cos\left(\theta-\text{90}\text{°}\right)=\sin\theta$

Therefore, $\sin\left(\theta-\text{90}\text{°}\right)=-\cos\theta$ and $\cos\left(\theta-\text{90}\text{°}\right)=\sin\theta$ .

Worked example 11: Co-functions

Express the following in terms of $t$ if $t = \sin \theta$ :

$\frac{\cos (\theta - \text{90}\text{°})\cos(\text{720}\text{°} + \theta) \tan(\theta - \text{360}\text{°})}{\sin^2(\theta + \text{360}\text{°}) \cos(\theta + \text{90}\text{°})}$

Simplify the expression using reduction formulae and co-functions

Use the CAST diagram to check in which quadrants the trigonometric ratios are positive and negative.

$\begin{align*} &\frac{\cos (\theta - \text{90}\text{°})\cos(\text{720}\text{°} + \theta) \tan(\theta - \text{360}\text{°})}{\sin^2(\theta + \text{360}\text{°}) \cos(\theta + \text{90}\text{°})} \\ =& \frac{\cos [- (\text{90}\text{°} - \theta)] \cos [2(\text{360}\text{°}) + \theta] \tan [-(\text{360}\text{°} - \theta)]}{\sin^2(\text{360}\text{°} + \theta) \cos(\text{90}\text{°} + \theta)} \\ =& \frac{\sin \theta \cos \theta \tan \theta }{\sin^2\theta (-\sin\theta)} \\ =& -\frac{\cos \theta \left( \frac{\sin \theta}{\cos \theta} \right)}{\sin^2\theta} \\ =& - \frac{1}{\sin \theta} \\ =& - \frac{1}{t} \end{align*}$

Co-functions

Textbook Exercise 6.5

$\dfrac{\cos(\text{90}\text{°} + \theta) \sin (\theta + \text{90}\text{°})}{\sin (-\theta)}$

\begin{align*} \dfrac{\cos(\text{90}\text{°} + \theta) \sin (\theta + \text{90}\text{°})}{\sin (-\theta)} &= \dfrac{-\sin\theta \cos\theta}{-\sin\theta} \\ &= \cos\theta \end{align*}

$\dfrac{2\sin(\text{90}\text{°} - x) + \sin (\text{90}\text{°} + x)}{\sin (\text{90}\text{°} - x) + \cos(\text{180}\text{°} + x)}$

\begin{align*} \dfrac{2\sin(\text{90}\text{°} - x) + \sin (\text{90}\text{°} + x)}{\sin (\text{90}\text{°} - x) + \cos(\text{180}\text{°} + x)} &=\dfrac{2\cos x+\cos x}{\cos x+\cos x} \\ &=\dfrac{3\cos x}{2\cos x} \\ &= \frac{3}{2} \end{align*}

$\sin \text{54}\text{°}$

\begin{align*} \sin \text{54}\text{°} &=\sin (\text{90}\text{°}-\text{36}\text{°}) \\ &=\cos \text{36}\text{°} \\ &=p \end{align*}

$\sin \text{36}\text{°}$

\begin{align*} \sin \text{36}\text{°} &= \sqrt{1 - \cos^2 \text{36}\text{°}} \\ &= \sqrt{1-p^2} \end{align*}

$\tan \text{126}\text{°}$

\begin{align*} \tan \text{126}\text{°} &=\frac{\sin(\text{90}\text{°}+\text{36}\text{°})}{\cos(\text{90}\text{°}+\text{36}\text{°})} \\ &=\frac{\cos\text{36}\text{°}}{-\sin\text{36}\text{°}} \\ &=-\frac{p}{\sqrt{1-p^2}} \end{align*}

$\cos \text{324}\text{°}$

\begin{align*} \cos \text{324}\text{°} &=\cos(\text{360}\text{°}-\text{36}\text{°}) \\ &=\cos\text{36}\text{°}\\ &=p \end{align*}

Reduction formulae and co-functions:

The reduction formulae hold for any angle $\theta$ . For convenience, we assume $\theta$ is an acute angle ( $\text{0}\text{°}<\theta <\text{90}\text{°}$ ).
When determining function values of ( $\text{180}\text{°}±\theta$ ), ( $\text{360}\text{°}±\theta$ ) and ( $-\theta$ ) the function does not change.
When determining function values of ( $\text{90}\text{°}±\theta$ ) and ( $\theta ±\text{90}\text{°}$ ) the function changes to its co-function.

second quadrant $(\text{180}\text{°}-\theta)$ or $(\text{90}\text{°}+\theta)$	first quadrant $(\theta)$ or $(\text{90}\text{°}-\theta)$
$\sin(\text{180}\text{°}-\theta)=+\sin\theta$	all trig functions are positive
$\cos(\text{180}\text{°}-\theta )=-\cos\theta$	$\sin(\text{360}\text{°}+\theta )=\sin\theta$
$\tan(\text{180}\text{°}-\theta )=-\tan\theta$	$\cos(\text{360}\text{°}+\theta )=\cos\theta$
$\sin(\text{90}\text{°}+\theta )=+\cos\theta$	$\tan(\text{360}\text{°}+\theta )=\tan\theta$
$\cos(\text{90}\text{°}+\theta )=-\sin\theta$	$\sin(\text{90}\text{°}-\theta )=\cos\theta$
	$\cos(\text{90}\text{°}-\theta )=\sin\theta$
third quadrant $(\text{180}\text{°}+\theta)$	fourth quadrant $(\text{360}\text{°}-\theta)$
$\sin(\text{180}\text{°}+\theta )=-\sin\theta$	$\sin(\text{360}\text{°}-\theta )=-\sin\theta$
$\cos(\text{180}\text{°}+\theta )=-\cos\theta$	$\cos(\text{360}\text{°}-\theta )=+\cos\theta$
$\tan(\text{180}\text{°}+\theta )=+\tan\theta$	$\tan(\text{360}\text{°}-\theta )=-\tan\theta$

Reduction formulae

Textbook Exercise 6.6

$A = \sin(\text{360}\text{°} - \theta) \cos (\text{180}\text{°} - \theta) \tan(\text{360}\text{°} + \theta)$

\begin{align*} A &= \sin(\text{360}\text{°} - \theta) \cos (\text{180}\text{°} - \theta) \tan(\text{360}\text{°} + \theta) \\ &= ( - \sin \theta )(- \cos \theta )( \tan \theta) \\ &= \sin \theta \cos \theta \frac{\sin \theta}{\cos \theta} \\ &= \sin^2 \theta \end{align*}

$B = \dfrac{\cos (\text{360}\text{°} + \theta) \cos(-\theta) \sin (-\theta)}{\cos (\text{90}\text{°} + \theta)}$

\begin{align*} B &= \dfrac{\cos (\text{360}\text{°} + \theta) \cos(-\theta) \sin (-\theta)}{\cos (\text{90}\text{°} + \theta)} \\ &= \dfrac{\cos \theta \cos \theta (- \sin \theta)}{- \sin \theta } \\ &= \dfrac{\cos^2 \theta \sin \theta}{\sin \theta } \\ &= \cos^2 \theta \\ &= \cos^2 \theta \end{align*}

$A + B = \ldots$

\begin{align*} A + B &= \cos^2 \theta + \sin^2 \theta \\ &= \text{1} \end{align*}

$\frac{A}{B} = \ldots$

\begin{align*} \frac{A}{B} &= \frac{\sin^2 \theta }{\cos^2 \theta} \\ &= \tan^2 \theta \end{align*}

$\sin \text{163}\text{°}$

\begin{align*} \sin \text{163}\text{°} &= \sin (\text{180}\text{°} - \text{17}\text{°})\\ &= \sin \text{17}\text{°} \end{align*}

$\cos \text{327}\text{°}$

\begin{align*} \cos \text{327}\text{°} &= \cos (\text{360}\text{°} - \text{33}\text{°})\\ &= \cos \text{33}\text{°} \end{align*}

$\tan \text{248}\text{°}$

\begin{align*} \tan \text{248}\text{°}&= \tan (\text{180}\text{°} + \text{68}\text{°})\\ &= \tan \text{68}\text{°} \end{align*}

$\cos (-\text{213}\text{°})$

\begin{align*} \cos (-\text{213}\text{°}) &= \cos \text{213}\text{°} \\ &= \cos (\text{180}\text{°} + \text{33}\text{°})\\ &= - \cos \text{33}\text{°} \end{align*}

$\dfrac{\sin(-\text{30}\text{°})}{\tan(\text{150}\text{°})} + \cos \text{330}\text{°}$

\begin{align*} \dfrac{\sin(-\text{30}\text{°})}{\tan(\text{150}\text{°})} + \cos \text{330}\text{°} &= \dfrac{- \sin \text{30}\text{°} }{\tan (\text{180}\text{°} - \text{30}\text{°})} + \cos (\text{360}\text{°} - \text{30}\text{°}) \\ &= \dfrac{- \sin \text{30}\text{°} }{- \tan \text{30}\text{°} } + \cos \text{30}\text{°} \\ &= \dfrac{ \sin \text{30}\text{°} }{ \frac{\sin \text{30}\text{°}}{\cos \text{30}\text{°}}} + \cos \text{30}\text{°} \\ &= \cos \text{30}\text{°} + \cos \text{30}\text{°} \\ &= 2 \cos \text{30}\text{°}\\ &= 2 \left( \frac{\sqrt{3}}{2} \right)\\ &= \sqrt{3} \end{align*}

$\tan \text{300}\text{°} \cos \text{120}\text{°}$

\begin{align*} \tan \text{300}\text{°} \cos \text{120}\text{°} &= \tan (\text{360}\text{°} - \text{60}\text{°}) \cos (\text{180}\text{°} - \text{60}\text{°}) \\ &= (-\tan \text{60}\text{°})(- \cos \text{60}\text{°}) \\ &= \left( \sqrt{3} \right) \left( \frac{1}{2} \right) \\ &= \frac{\sqrt{3}}{2} \end{align*}

$(1 - \cos \text{30}\text{°})(1 - \cos \text{210}\text{°})$

\begin{align*} (1 - \cos \text{30}\text{°})(1 - \cos \text{210}\text{°}) &= (1 - \cos \text{30}\text{°})(1 - \cos (\text{180}\text{°} + \text{30}\text{°})) \\ &= (1 - \cos \text{30}\text{°})(1 + \cos \text{30}\text{°}) \\ &= 1 - \cos^2 \text{30}\text{°} \\ &= \sin^2 \text{30}\text{°} \\ &= \sin^2 \text{30}\text{°} \\ &= \left( \frac{1}{2} \right)^2 \\ &= \frac{1}{4} \end{align*}

$\cos \text{780}\text{°} - (\sin \text{315}\text{°})(\cos \text{405}\text{°})$

\begin{align*} &\cos \text{780}\text{°} - (\sin \text{315}\text{°})(\cos \text{405}\text{°}) \\ &= \cos (2(\text{360}\text{°}) + \text{60}\text{°}) - (\sin (\text{360}\text{°} -\text{45}\text{°}))(\cos (\text{360}\text{°} + \text{45}\text{°})) \\ &= \cos\text{60}\text{°} + \sin \text{45}\text{°} \cos\text{45}\text{°} \\ &= \left( \frac{1}{2} \right) + \left( \frac{1}{\sqrt{2}} \right)\left( \frac{1}{\sqrt{2}} \right) \\ &= \left( \frac{1}{2} \right) + \left( \frac{1}{2} \right) \\ &= \text{1} \end{align*}

Prove that the following identity is true and state any restrictions:

$\dfrac{\sin(\text{180}\text{°} + \alpha) \tan(\text{360}\text{°} + \alpha) \cos \alpha}{\cos(\text{90}\text{°} - \alpha)} = \sin \alpha$

\begin{align*} \text{LHS } &= \dfrac{\sin(\text{180}\text{°} + \alpha) \tan(\text{360}\text{°} + \alpha) \cos \alpha}{\cos(\text{90}\text{°} - \alpha)} \\ &= \dfrac{(-\sin \alpha) \tan \alpha \cos \alpha}{\sin \alpha } \\ &= -\frac{\sin \alpha}{\cos \alpha} \cos \alpha \\ &= \sin \alpha \\ &= \text{RHS} \end{align*}

6.2 Trigonometric identities

Table of Contents

6.4 Trigonometric equations

6.3 Reduction formula (EMBHJ)

Deriving reduction formulae (EMBHK)

Reduction formulae for function values of 180°±θ\text{180}\text{°}±\theta

Worked example 7: Reduction formulae for function values of 180°±θ\text{180}\text{°} \pm \theta

Use reduction formulae to write the trigonometric function values in terms of acute angles and θ\theta

Simplify

Reduction formulae for function values of \(\text{180}\text{°} \pm \theta\)

Reduction formulae for function values of (360°±θ\text{360}\text{°} \pm \theta) and (−θ)(- \theta)

Worked example 8: Reduction formulae for function values of 360°±θ\text{360}\text{°} \pm \theta

Using reduction formula

Worked example 9: Using reduction formula

Simplify the expression using reduction formulae and special angles

Using reduction formula

Reduction formulae for function values of 90°±θ\text{90}\text{°}±\theta

Worked example 10: Using the co-function rule

Worked example 11: Co-functions

Simplify the expression using reduction formulae and co-functions

Co-functions

Reduction formulae

Reduction formulae for function values of $\text{180}\text{°}±\theta$

Worked example 7: Reduction formulae for function values of $\text{180}\text{°} \pm \theta$

Use reduction formulae to write the trigonometric function values in terms of acute angles and $\theta$

Reduction formulae for function values of ( $\text{360}\text{°} \pm \theta$ ) and $(- \theta)$

Worked example 8: Reduction formulae for function values of $\text{360}\text{°} \pm \theta$

Reduction formulae for function values of $\text{90}\text{°}±\theta$