\((3;7)\) and \((-6;1)\)
4.2 Equation of a line
|
Previous
4.1 Revision
|
Next
4.3 Inclination of a line
|
4.2 Equation of a line (EMBG8)
We can derive different forms of the straight line equation. The different forms are used depending on the information provided in the problem:
- The two-point form of the straight line equation: y−y1x−x1=y2−y1x2−x1
- The gradient–point form of the straight line equation: y−y1=m(x−x1)
- The gradient–intercept form of the straight line equation: y=mx+c
The two-point form of the straight line equation (EMBG9)

Given any two points (x1;y1) and (x2;y2), we can determine the equation of the line passing through the two points using the equation:
y−y1x−x1=y2−y1x2−x1
Worked example 3: The two-point form of the straight line equation
Find the equation of the straight line passing through P(−1;−5) and Q(5;4).
Draw a sketch

Assign variables to the coordinates of the given points
Let the coordinates of P be (x1;y1) and Q(x2;y2)
x1=−1;y1=−5;x2=5;y2=4
Write down the two-point form of the straight line equation
y−y1x−x1=y2−y1x2−x1
Substitute the values and make y the subject of the equation
y−(−5)x−(−1)=4−(−5)5−(−1)y+5x+1=96y+5=32(x+1)y+5=32x+32y=32x−72
Write the final answer
y=32x−312
The two-point form of the straight line equation
Determine the equation of the straight line passing through the points:
\((1;-\frac{11}{4})\) and \((\frac{2}{3}; -\frac{7}{4})\)
\((-2;1)\) and \((3;6)\)
\((2;3)\) and \((3;5)\)
\((1;-5)\) and \((-7;-5)\)
\((-4;0)\) and \((1;\frac{15}{4})\)
\((s;t)\) and \((t;s)\)
\((-2;-8)\) and \((1;7)\)
\((2p;q)\) and \((0;-q)\)
The gradient–point form of the straight line equation (EMBGB)
We derive the gradient–point form of the straight line equation using the definition of gradient and the two-point form of a straight line equation y−y1x−x1=y2−y1x2−x1
Substitute m=y2−y1x2−x1 on the right-hand side of the equation y−y1x−x1=m
Multiply both sides of the equation by (x−x1) y−y1=m(x−x1)
To use this equation, we need to know the gradient of the line and the coordinates of one point on the line.
Worked example 4: The gradient–point form of the straight line equation
Determine the equation of the straight line with gradient m=−13 and passing through the point (−1;1).
Draw a sketch
We notice that m<0, therefore the graph decreases as x increases.
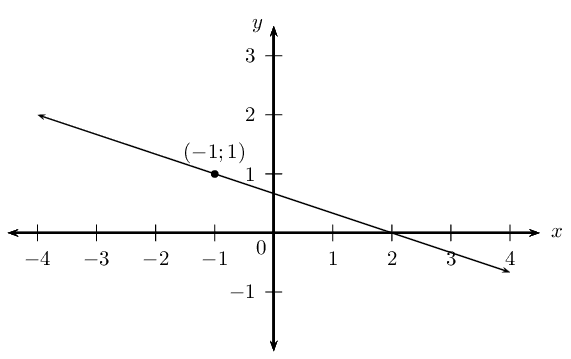
Write down the gradient–point form of the straight line equation
y−y1=m(x−x1)
Substitute the value of the gradient
y−y1=−13(x−x1)
Substitute the coordinates of the given point
y−1=−13(x−(−1))y−1=−13(x+1)y=−13x−13+1=−13x+23
Write the final answer
The equation of the straight line is y=−13x+23.
If we are given two points on a straight line, we can also use the gradient–point form to determine the equation of a straight line. We first calculate the gradient using the two given points and then substitute either of the two points into the gradient–point form of the equation.
Worked example 5: The gradient–point form of the straight line equation
Determine the equation of the straight line passing through (−3;2) and (5;8).
Draw a sketch
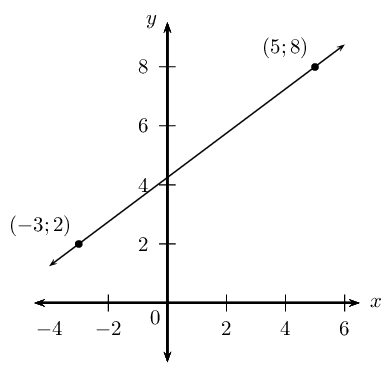
Assign variables to the coordinates of the given points
x1=−3;y1=2;x2=5;y2=8
Calculate the gradient using the two given points
m=y2−y1x2−x1=8−25−(−3)=68=34
Write down the gradient–point form of the straight line equation
y−y1=m(x−x1)
Substitute the value of the gradient
y−y1=34(x−x1)
Substitute the coordinates of a given point
y−y1=34(x−x1)y−2=34(x−(−3))y−2=34(x+3)y=34x+94+2=34x+174
Write the final answer
The equation of the straight line is y=34x+414.
Gradient–point form of a straight line equation
Determine the equation of the straight line:
passing through the point \((-1;\frac{10}{3})\) and with \(m=\frac{2}{3}\).
with \(m= -1\) and passing through the point \((-2;0)\).
passing through the point \((3;-1)\) and with \(m=-\frac{1}{3}\).
parallel to the \(x\)-axis and passing through the point \((0;11)\).
passing through the point \((1;5)\) and with \(m=-2\).
perpendicular to the \(x\)-axis and passing through the point \((-\frac{3}{2};0)\).
with \(m = -\text{0,8}\) and passing through the point \((10;-7)\).
with undefined gradient and passing through the point \((4;0)\).
with \(m= 3a\) and passing through the point \((-2;-6a+b)\).
The gradient–intercept form of a straight line equation (EMBGC)
Using the gradient–point form, we can also derive the gradient–intercept form of the straight line equation.
Starting with the equation
y−y1=m(x−x1)
Expand the brackets and make y the subject of the formula
y−y1=mx−mx1y=mx−mx1+y1y=mx+(y1−mx1)We define constant c such that c=y1−mx1 so that we get the equation
y=mx+c
This is also called the standard form of the straight line equation.
Notice that when x=0, we have
y=m(0)+c=cTherefore c is the y-intercept of the straight line.
Worked example 6: The gradient–intercept form of straight line equation
Determine the equation of the straight line with gradient m=−2 and passing through the point (−1;7).
Slope of the line
We notice that m<0, therefore the graph decreases as x increases.
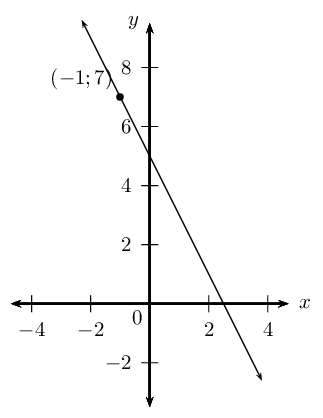
Write down the gradient–intercept form of straight line equation
y=mx+c
Substitute the value of the gradient
y=−2x+c
Substitute the coordinates of the given point and find c
y=−2x+c7=−2(−1)+c7−2=c∴c=5This gives the y-intercept (0;5).
Write the final answer
The equation of the straight line is y=−2x+5.
If we are given two points on a straight line, we can also use the gradient–intercept form to determine the equation of a straight line. We solve for the two unknowns m and c using simultaneous equations — using the methods of substitution or elimination.
Worked example 7: The gradient–intercept form of straight line equation
Determine the equation of the straight line passing through the points (−2;−7) and (3;8).
Draw a sketch
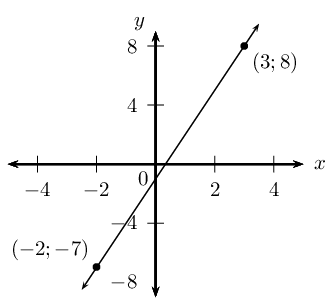
Write down the gradient–intercept form of straight line equation
y=mx+c
Substitute the coordinates of the given points
−7=m(−2)+c−7=−2m+c…(1)8=m(3)+c8=3m+c…(2)We have two equations with two unknowns; we can therefore solve using simultaneous equations.
Make the coefficient of one of the variables the same in both equations
We notice that the coefficient of c in both equations is 1, therefore we can subtract one equation from the other to eliminate c:
−7=−2m+c−(8=3m+c)−15=−5m∴3=mSubstitute m=3 into either of the two equations and determine c: −7=−2m+c−7=−2(3)+c∴c=−1
Write the final answer
The equation of the straight line is y=3x−1.
The gradient–intercept form of a straight line equation
Determine the equation of the straight line:
passing through the point \((\frac{1}{2};4)\) and with \(m=2\).
passing through the points \((\frac{1}{2};-2)\) and \((2;4)\).
passing through the points \((2;-3)\) and \((-1;0)\).
passing through the point \((2;-\frac{6}{7})\) and with \(m=-\frac{3}{7}\).
which cuts the \(y\)-axis at \(y=-\frac{1}{5}\) and with \(m=\frac{1}{2}\).
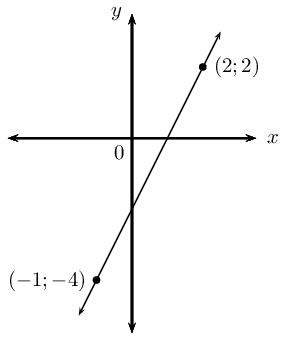

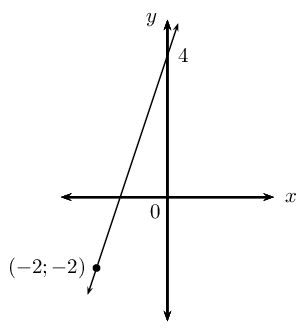
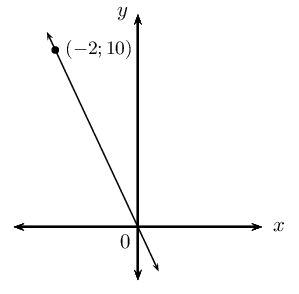
|
Previous
4.1 Revision
|
Table of Contents |
Next
4.3 Inclination of a line
|
