Chapter 12: Two-dimensional shapes
12.1 Types and properties of shapes
A two-dimensional (2D) shape has two dimensions that we can measure: we can measure the length and we can measure the breadth (sometimes called the width) of the shape. For example, a square is a 2D shape. All the sides of a square are straight lines. A circle is also a 2D shape, but a circle has a curved edge.
We use a special name for the 2D shapes that are formed by straight lines. They are called polygons. We name polygons by counting the number of straight sides that they have. A polygon with five sides is called a pentagon and a polygon with six sides is a hexagon.
There is a whole group of polygons with four sides, and they are the quadrilaterals. You have worked with squares, rectangles, parallelograms, rhombuses and trapeziums before. All of them are examples of quadrilaterals.
polygon A polygon is a closed flat shape with three or more straight sides.
quadrilateral A quadrilateral is a polygon that has four sides and four angles.
Properties of two-dimensional shapes
The properties of these two-dimensional shapes are listed below.
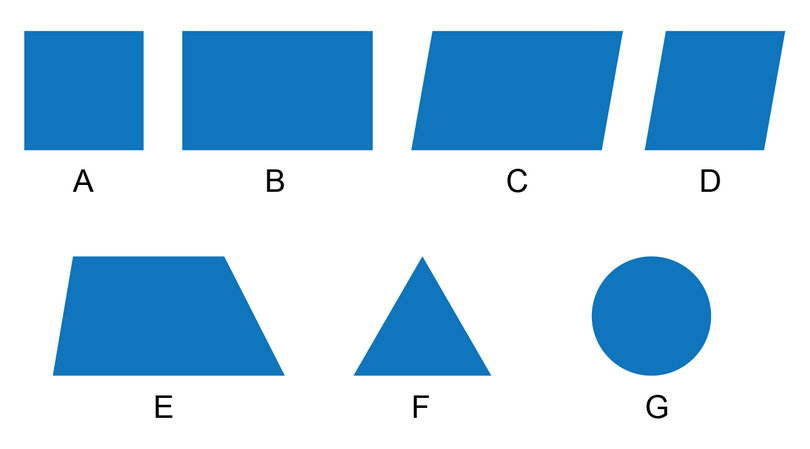
A. Square
- A square has four equal sides and four right angles.
- The opposite sides of a square are parallel.
B. Rectangle
- A rectangle has two pairs of equal sides. The longer side of a rectangle is the length and the shorter side is the breadth.
- A rectangle has four right angles.
- The opposite sides of a rectangle are parallel.
C. Parallelogram
- A parallelogram has two pairs of opposite sides that are parallel and equal.
- The opposite angles are equal.

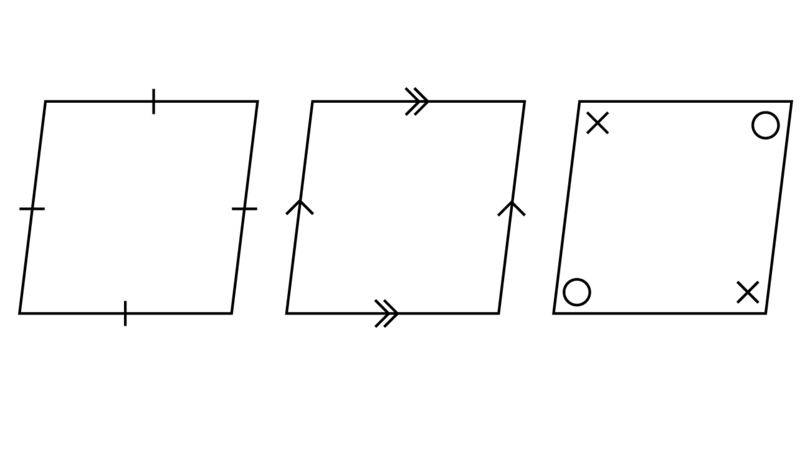
D. Rhombus
- A rhombus has four equal sides.
- Opposite sides are parallel.
- The opposite angles are equal.
- Two of the angles are acute (more closed) angles, and two are obtuse (more open) angles.
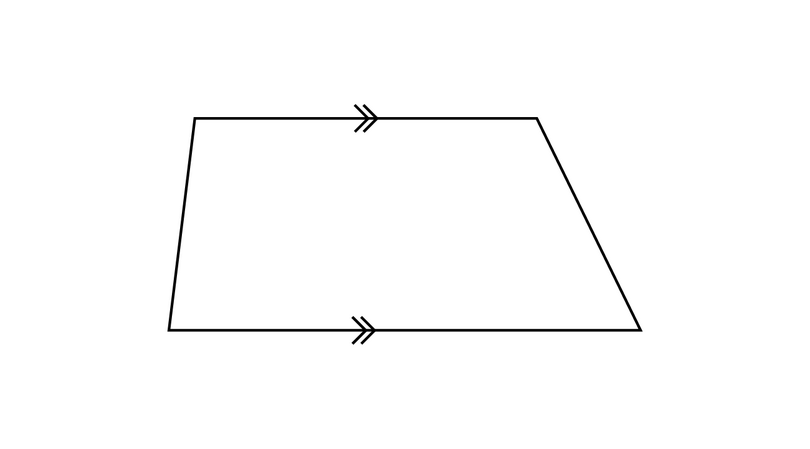
E. Trapezium
- A trapezium has one pair of parallel sides.
- The sides may all be different in length.
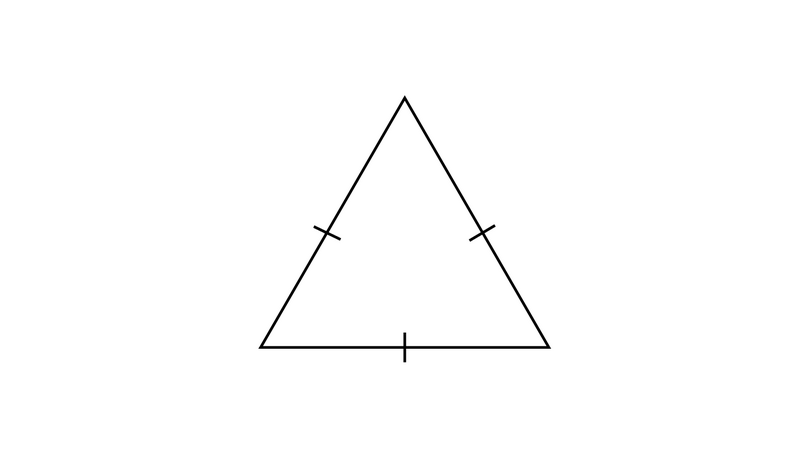
F. Triangle
- A triangle has three sides and three angles. All the sides and angles can be different sizes.
- There are different types of triangles. The triangle shown is an equilateral triangle, which has all three sides equal in length and all three angles equal.
G. Circle
- The properties and different parts of a circle are explained further on.
Worked example 12.1: Comparing two-dimensional shapes
Look at the two shapes. State which shape is NOT a polygon, and identify and name the quadrilateral.

-
Step 1: Remember the definition of a polygon.
A polygon is a closed flat shape with three or more straight sides.
-
Step 2: Identify the shape without straight sides.
Answer: The circle has curved sides, so it is not a polygon.
-
Step 3: Remember the definition of a quadrilateral.
A quadrilateral is a polygon that has four sides and four angles.
-
Step 4: Identify the shape that has four sides and four angles, and name the shape.
Answer: The quadrilateral is a trapezium.
Exercise 12.1: Compare two-dimensional shapes
-
Compare a square and a rectangle. What is similar? What is different?
Similar: A square has four right angles. A rectangle also has four right angles.
Different: A square has four equal sides. A rectangle has two longer sides and two shorter sides.
-
Compare a square and a rhombus. What is similar? What is different?
Similar: A square has four equal sides. A rhombus also has four equal sides.
Different: A square has four right angles. A rhombus has two acute angles and two obtuse angles.
-
Compare a trapezium and a parallelogram. What is similar? What is different?
Similar: A trapezium is a quadrilateral. A parallelogram is also a quadrilateral.
Different: A trapezium has one pair of parallel sides. A parallelogram has two pairs of parallel sides.
-
Compare a parallelogram and a rhombus. What is similar? What is different?
Similar: A parallelogram has two pairs of parallel sides. A rhombus also has two pairs of parallel sides.
Different: A parallelogram has two longer sides and two shorter sides. A rhombus has four equal sides.
-
Compare a triangle and a square. What is similar? What is different?
Similar: A triangle is a polygon. A square is also a polygon.
Different: A triangle has three sides. A square has four sides.
Parts and properties of a circle
A circle is a 2D shape with a curved edge, so a circle is not a polygon. We use special names for the different parts of a circle.
- The perimeter of a circle (the length around the circle) is called its circumference.
- A line segment that goes all the way across the circle and passes through the centre is called a diameter.
- A line segment from the centre of the circle to a point on the circumference is called a radius.
Worked example 12.2: Identifying parts of a circle
In the diagram below, O is the centre of the circle. T, S, and V are labels for parts of the circle. Which of the labels identifies the circumference of the circle?
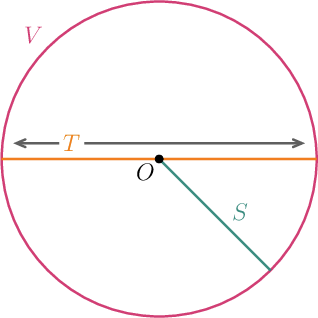
-
Step 1: Identify the parts of the circle.
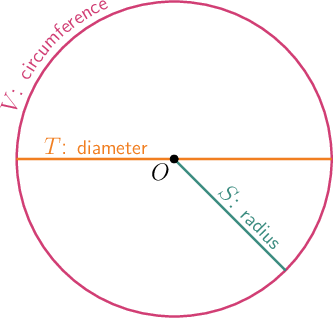
A line segment that goes all the way across the circle and passes through the centre is called a diameter.
A line segment from the centre of the circle to a point on the circumference is called a radius.
The perimeter of a circle (the length around the circle) is called its circumference.
-
Step 2: Read the question again and give the correct answer.
V is the circumference of the circle.
Exercise 12.2: Identify parts of a circle
-
In the diagram below, O is the centre of the circle. T, S and V are labels for parts of the circle.
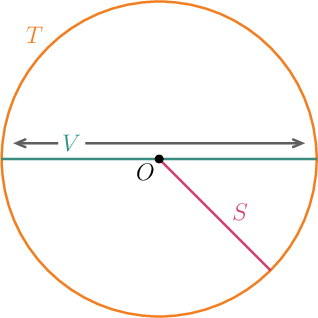
- Which of the labels identifies a diameter of the circle?
V is a diameter of the circle.
- Which of the labels identifies a radius of the circle?
S is a radius of the circle.
- Which of the labels identifies the circumference of the circle?
T is the circumference of the circle.
-
A diagram of a circle is given. O is the centre of the circle.
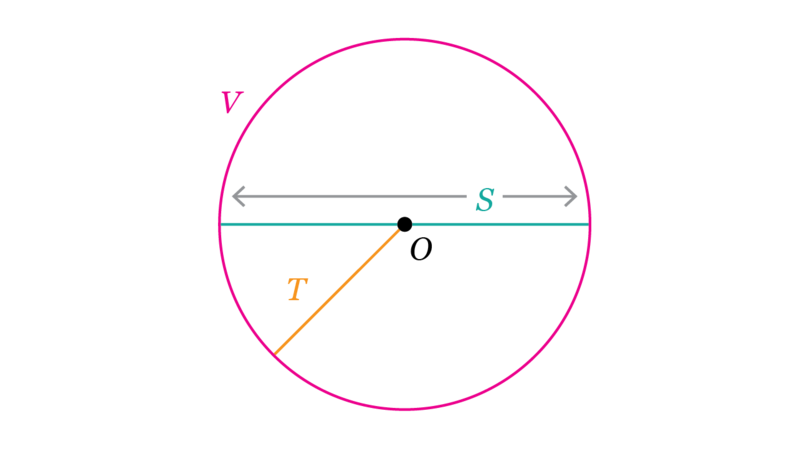
- Which part of the circle is labelled T?
T is a line segment from the centre of the circle to a point on the circumference, so T is a radius.
- Which part of the circle is labelled S?
S is a line segment that goes all the way across the circle and passes through the centre, so S is a diameter.
- Which part of the circle is labelled V?
V shows the perimeter of the circle (the length around the circle), so V is the circumference.
-
Select a word from Column B that best fits the description in Column A.
Column A Column B a) A line segment that goes all the way across the circle and passes through the centre. A: Circumference b) The perimeter of a circle (the length around the circle). B: Radius c) A line segment from the centre of the circle to a point on the circumference. C: Diameter - C
- A
- B
12.2 Perimeter of two-dimensional shapes
If you trace your finger around the edge of a book or a plate, you have traced the perimeter of the shape with your finger. The perimeter of a football field is the white line around the entire field.
Perimeter is a distance, so it is measured in units of length (such as mm, cm, m or km).
perimeter Perimeter is the distance around the outside of a shape.
Counting steps to find perimeter
We can use a practical method to find the perimeter of a 2D shape if the shape is drawn on a grid.
Because perimeter is the distance around the outside of a shape, to find the perimeter of a polygon, we add up the lengths of all of the sides as if we are walking all the way around the edge of the shape. It is important to begin and end at the same corner of the shape.
Worked example 12.3: Finding the perimeter of a square
Determine the perimeter of the square shown in the diagram by counting the steps.
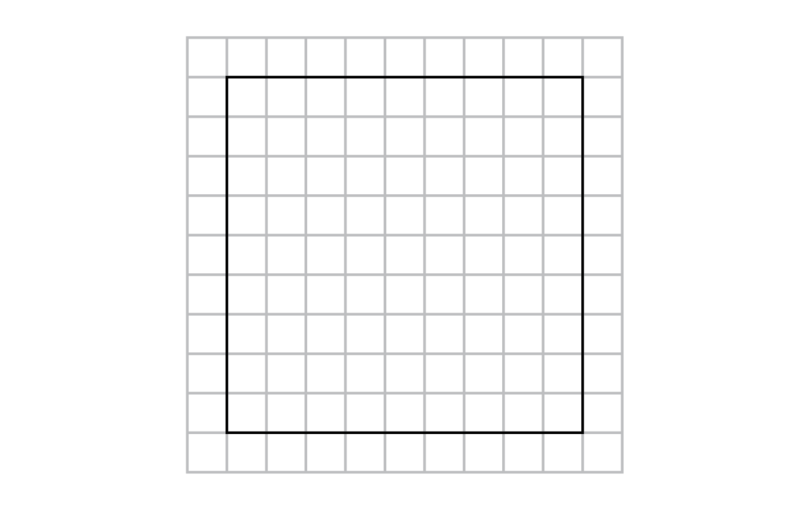
-
Step 1: Begin to walk at Point J and follow the arrows to walk around the shape. Count each step as you walk.
Number of steps for blue arrow = 9 steps
Number of steps for pink arrow = 9 steps
Number of steps for green arrow = 9 steps
Number of steps for red arrow = 9 steps
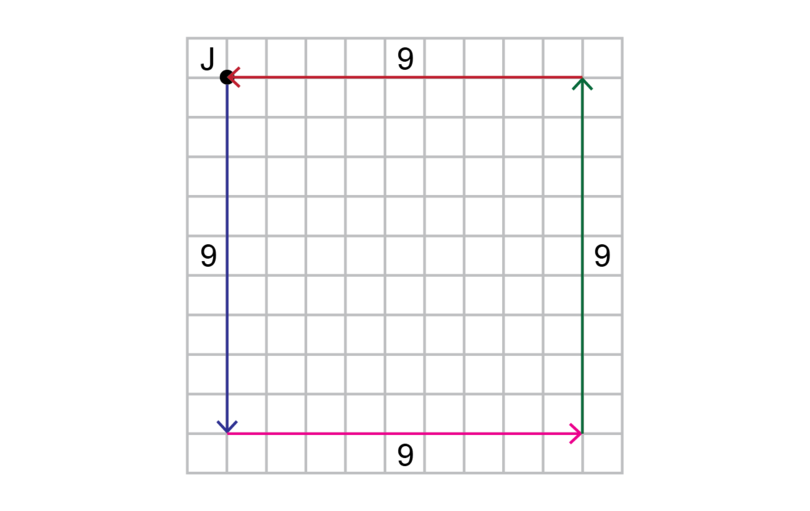
-
Step 2: Calculate the total number of steps that you have taken.
Perimeter=9+9+9+9=36 stepsTotal number of steps = 36 steps
-
Step 3: Give the answer
The distance around the shape is 36 steps, so the perimeter of the square is 36 steps.
Worked example 12.4: Finding the perimeter of a rectangle
Determine the perimeter of the rectangle provided by counting the steps. The length of one side of each small square represents 1 km.
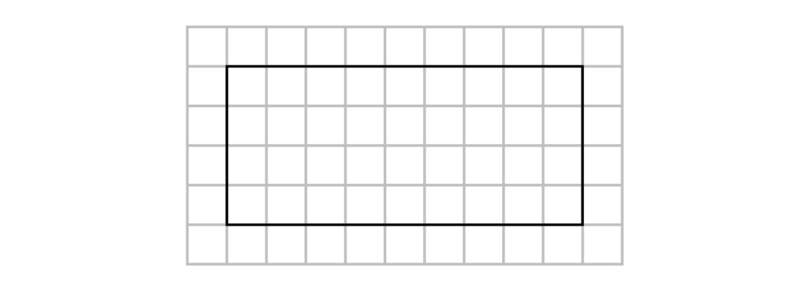
-
Step 1: Begin to walk at Point J and follow the arrows to walk around the shape. Count each step as you walk.
Number of steps for red arrow = 9 steps
Number of steps for blue arrow = 4 steps
Number of steps for green arrow = 9 steps
Number of steps for pink arrow = 4 steps
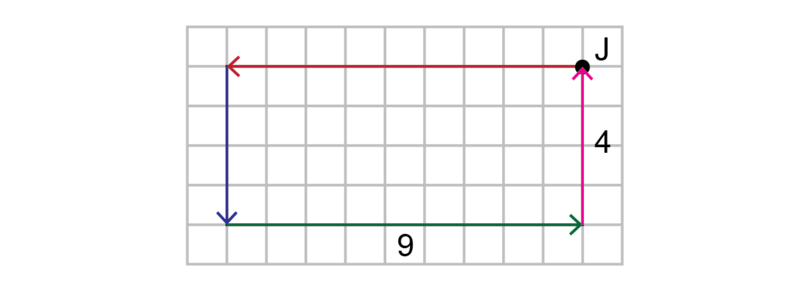
-
Step 2: Calculate the total number of steps that you have taken.
Perimeter=9+4+9+4=26 stepsTotal number of steps = 26 steps
-
Step 3: Give the answer.
The distance around the shape is 26 steps, so the perimeter of the rectangle is 26 km.
Exercise 12.3: Count steps to find perimeter
-
Find the perimeter the rectangle given below by using the grid on which it is plotted. The length of one side of each small square represents 1 km.
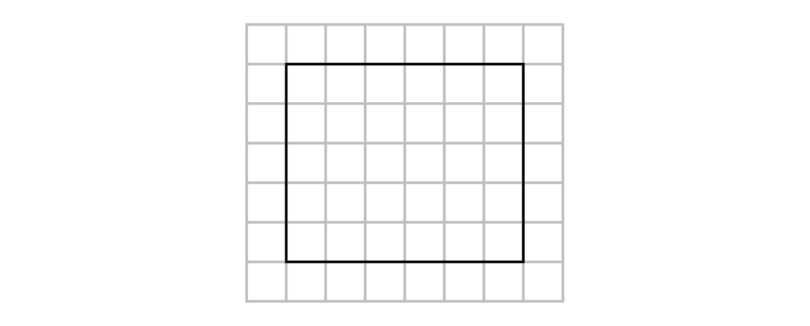
To find the perimeter of a polygon, we add up all of the sides as if we are walking around the edge of the shape.
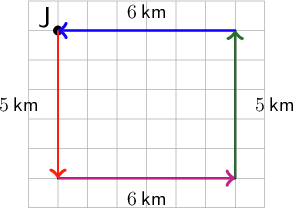
If we start at Point J and walk around the shape, we get:
Perimeter=5+6+5+6=22 kmTotal number of steps = 22 steps
The distance around the shape is 22 steps, so the perimeter of the rectangle is 22 km.
-
Find the perimeter of the following shape. Begin at the dot and follow the direction shown by the arrow. The length of one side of each small square represents 1 cm.
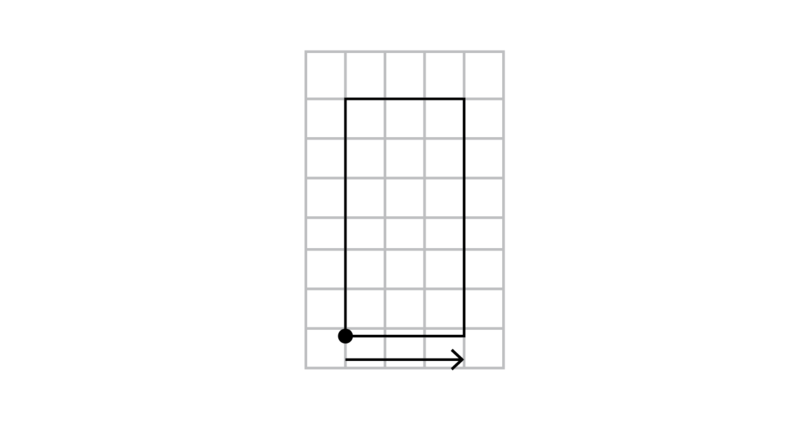
To find the perimeter of a polygon, we add up all of the sides as if we are walking around the edge of the shape.
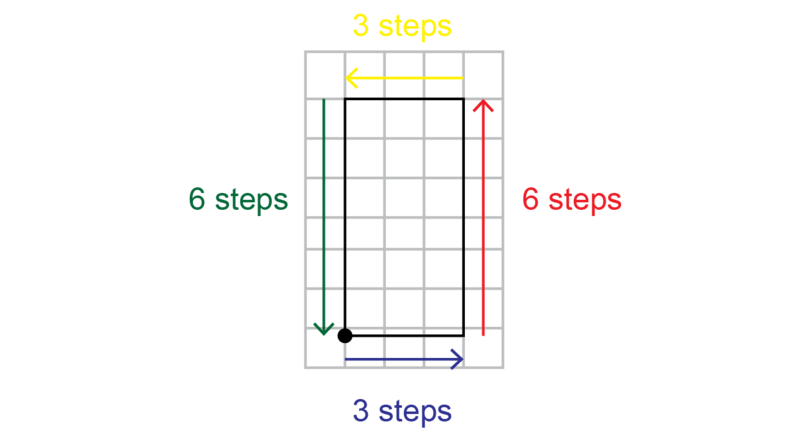
If we start at the dot and walk around the shape, we get:
Perimeter=3+6+3+6=18 cmTotal number of steps = 18 steps
The perimeter of the shape is 18 cm.
-
Find the perimeter of this shape. The length of one side of each small square is 1 cm.
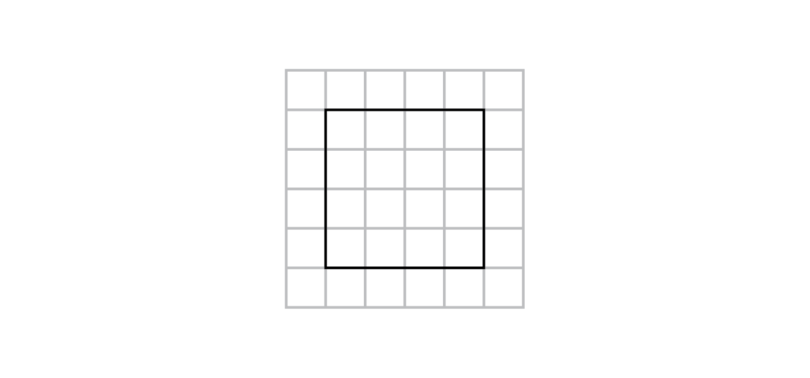
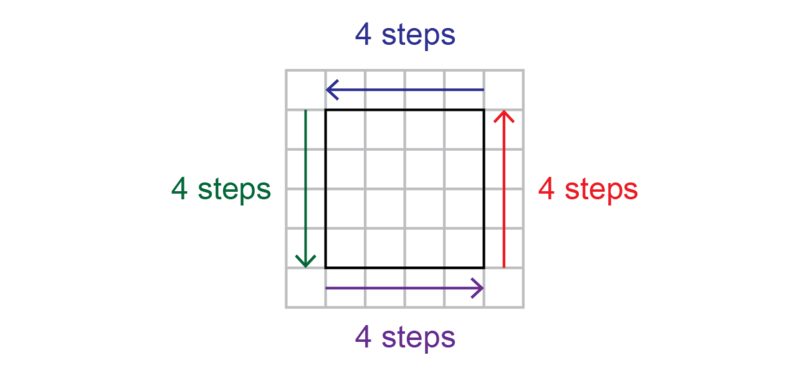
Number of steps for red arrow = 4 steps
Number of steps for purple arrow = 4 steps
Number of steps for green arrow = 4 steps
Number of steps for pink arrow = 4 steps
Perimeter=4+4+4+4=16 stepsTotal number of steps = 16 steps
The perimeter of the square is 16 cm.
-
Find the perimeter of this shape. The length of one side of each small square is 1 km.
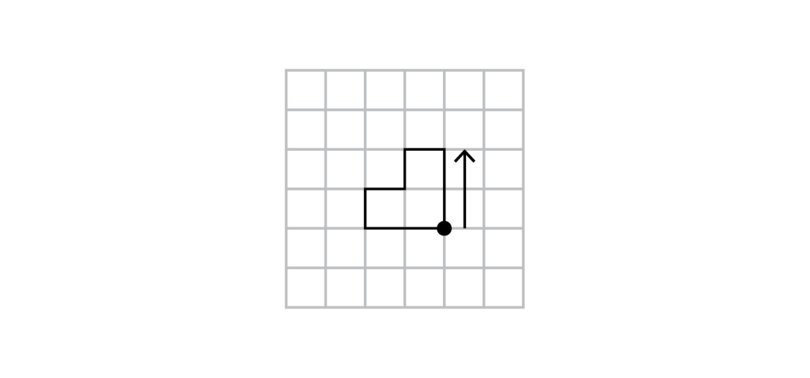
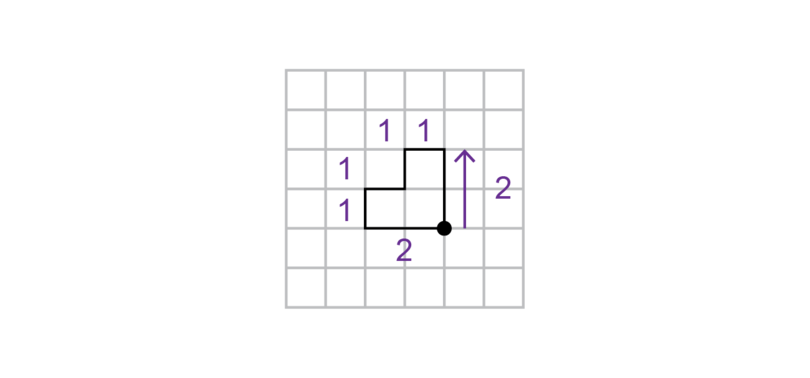 Sum of steps=2+1+1+1+1+2
=8 steps
Sum of steps=2+1+1+1+1+2
=8 steps
The distance around the shape is 8 steps.
The perimeter of the shape is 8 km.
-
Find the perimeter of this shape. The length of one side of each small square is 1 cm.
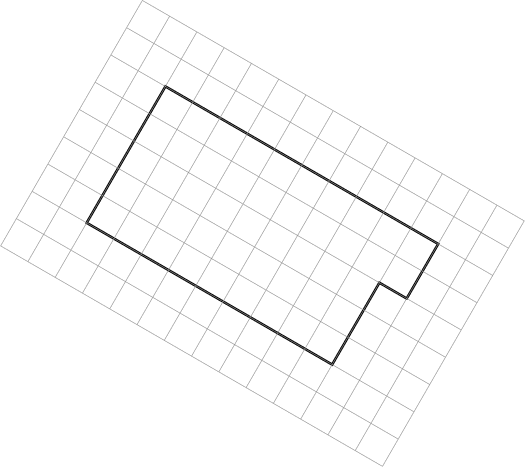
Perimeter=5 steps across+10 steps down+2 steps across+1 step up+3 steps across+9 steps up=5+10+2+1+3+9=30 steps
The perimeter is 30 cm.
-
Find the perimeter the shape. The length of one side of each small square is 1 m.
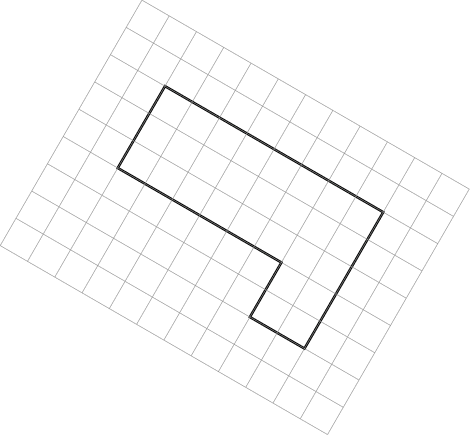
Perimeter=8 steps across+5 steps up+2 steps across+2 steps down+6 steps across+3 steps down=8+5+2+2+6+3=26 steps
The perimeter is 26 m.
Perimeter of regular polygons
If a shape is not drawn on grid paper, it will not be possible to "walk" around the shape and count the steps to find the perimeter. Then, to find the perimeter of a polygon, we need to know the lengths of all the sides of the shape.
When working out the perimeter, it is important to look at a diagram and to check whether or not it is a regular polygon that you are working with.
regular polygon In a regular polygon, all the sides have the same length and all the angles are equal.
perimeter of a polygon The perimeter of any polygon is the sum of the lengths of all the sides.
Units of measurement for lengths
Perimeter is a distance, and is measured using units of length, such as mm, cm, m or km.
- Millimetres (mm) and centimetres (cm) are appropriate for measuring short lengths. They are used on your ruler.
-
Metres (m) are longer. One metre is about the length of one big step, or three rulers placed end to end. There are 100 centimetres in one metre.
100 cm = 1 m

-
Kilometres (km) are good for measuring longer distances. It takes about 20 minutes to walk one kilometre (if you are walking at a relaxed speed). There are 1,000 metres in one kilometre.
1,000 m = 1 km
Worked example 12.5: Calculating perimeter of a regular polygon
Calculate the perimeter of the regular polygon shown in the diagram. The length of one side from W to V is 12 millimetres.
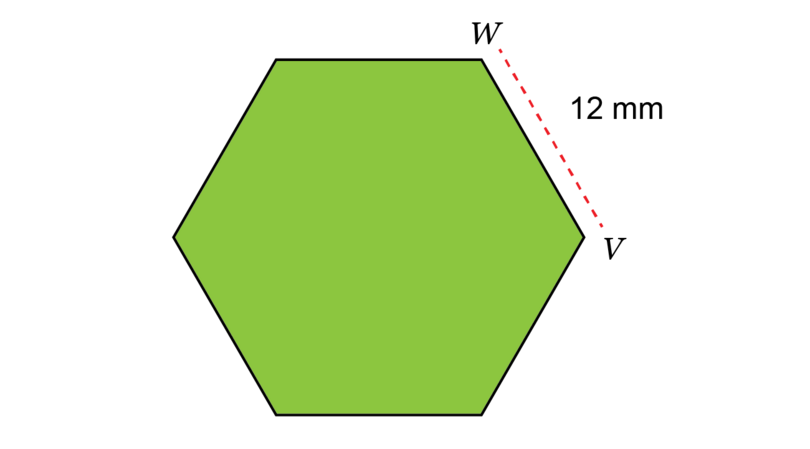
-
Step 1: Check whether the shape is a regular polygon.
If the instruction tells you that the diagram shows a regular polygon, then you can take this as a given. If you are not told, you can measure. This shape has six equal sides and six equal angles, so it is a regular polygon (named a regular hexagon).
-
Step 2: Work out what calculation to do.
You know that one side is 12 mm. So you need to multiply the number of sides by 12 mm.
-
Step 3: Do the calculation, and remember to write the correct units in the answer.
Perimeter=6×12 mm=72 mm
As you have seen in the worked example above, we can use a formula to calculate the perimeter of a regular polygon, because all the sides have the same lengths.
Perimeter of regular polygon = number of sides×length of one sideExercise 12.4 Calculate perimeter of regular polygons
-
Calculate the perimeter of the regular polygon below. The length of one side is 7 centimetres.
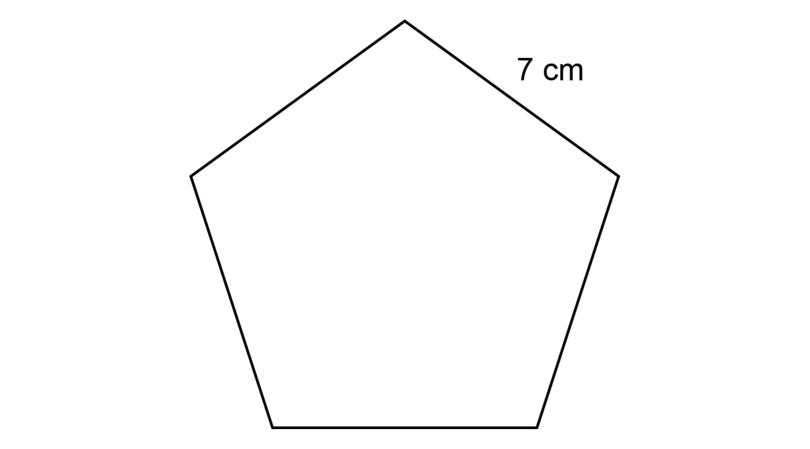
It is stated that the shape is a regular polygon, so the shape has equal sides. There are 5 sides, and the length of one side is 7 cm.
Perimeter=number of sides×length of one side=5×7 cm=35 cm -
Calculate the perimeter of the regular polygon below. The length of one side is 4.5 metres.

The shape has 4 equal sides, and the length of one side of the square is 4.5 m.
Perimeter=number of sides×length of one side=4×4.5 m=18 m -
Calculate the perimeter of the regular polygon below. The length of one side is 20 centimetres.
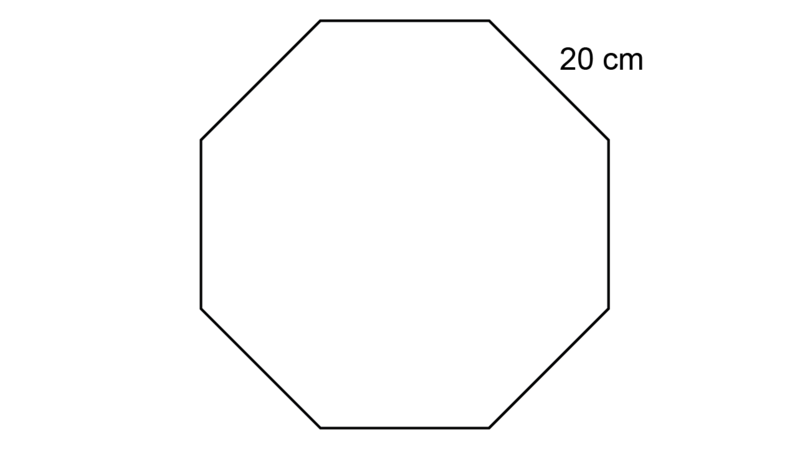
The shape has 8 equal sides, and the length of one side is 20 cm.
Perimeter=number of sides×length of one side=8×20 cm=160 cm -
Calculate the perimeter of the regular polygon below. The length of one side from W to V is 2.6 kilometres.

The shape has 10 equal sides, and the length of one side of the regular decagon is 2.6 km.
Perimeter=number of sides×length of one side=10×2.6 km=26 km -
Calculate the perimeter of the regular polygon below. The length of one side is 30 millimetres.
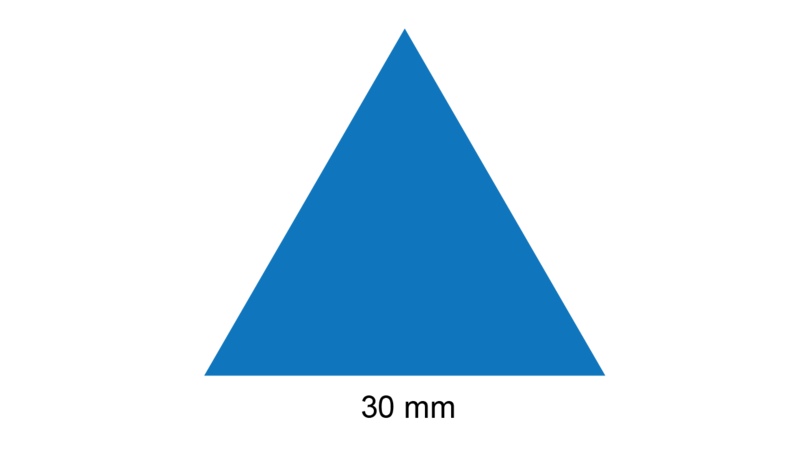
The shape has 3 equal sides, and the length of one side of the equilateral triangle is 30 mm.
\begin {align} \text{Perimeter} &= \text{number of sides} \times \text{length of one side}\\ &= 3 \times \text{30 mm}\\ &= \text{90 mm} \end{align}
Perimeter of irregular polygons
All polygons are not regular polygons. It is possible to draw a polygon where each side has a different length. It is also possible to draw a polygon where some of the sides are equal in length, but others have different lengths.
irregular polygon In an irregular polygon, all the sides are not the same length and all the angles are not equal.
To determine the perimeter of an irregular polygon, we have to find the sum of the lengths of all the sides. We cannot use a formula, so we simply add the lengths of all the sides.
Worked example 12.6: Calculating perimeter of an irregular triangle
Find the perimeter of the triangle given below.
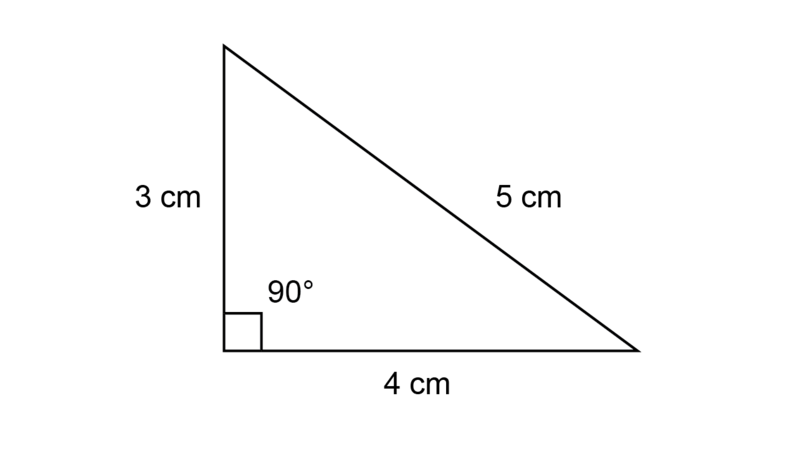
-
Step 1: Look at the diagram and take note of the information given.
The triangle is not a regular polygon. Each side of the triangle has a different length.
-
Step 2: Think of how you "walked" around a polygon. Write down the calculation that you must do.
Perimeter=3 cm+4 cm+5 cm -
Step 3: Do the calculation, and remember to write the correct units in the answer.
\begin {align} \text{Perimeter} &= \text{3 cm} + \text{4 cm} + \text{5 cm}\\ &= \text{12 cm} \end {align}
Worked example 12.7: Calculating perimeter of a parallelogram
Find the perimeter of the parallelogram given below.

-
Step 1: Look at the diagram and take note of the information given.
In a parallelogram, the opposite sides have equal lengths. Fill in the missing lengths.

-
Step 2: Think of how you "walked" around a polygon. Write down the calculation that you must do.
Perimeter=20 mm+7 mm+20 mm+7 mm -
Step 3: Do the calculation, writing the units as part of the answer.
Perimeter=20 mm+7 mm+20 mm+7 mm=54 mm
Exercise 12.5: Calculate perimeter of irregular polygons
-
Give the name of the irregular polygon shown in the diagram, and calculate its perimeter.
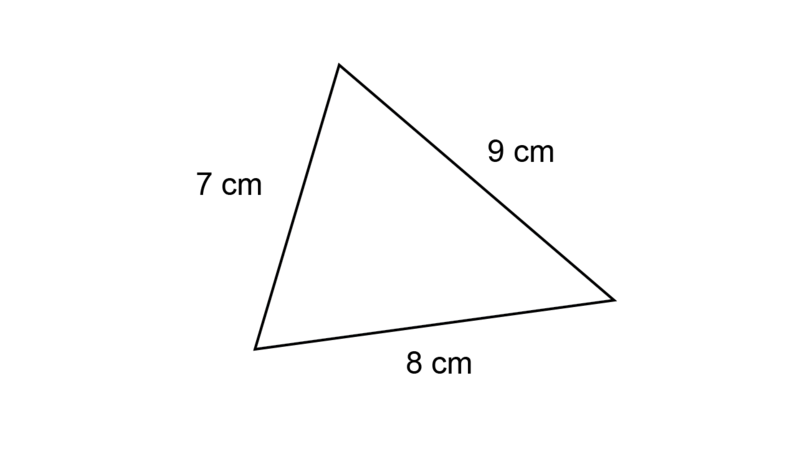
Perimeter=7 cm+8 cm+9 cm=24 cm
The name of the polygon: triangle.
-
Give the name of the irregular polygon shown in the diagram, and find its perimeter.
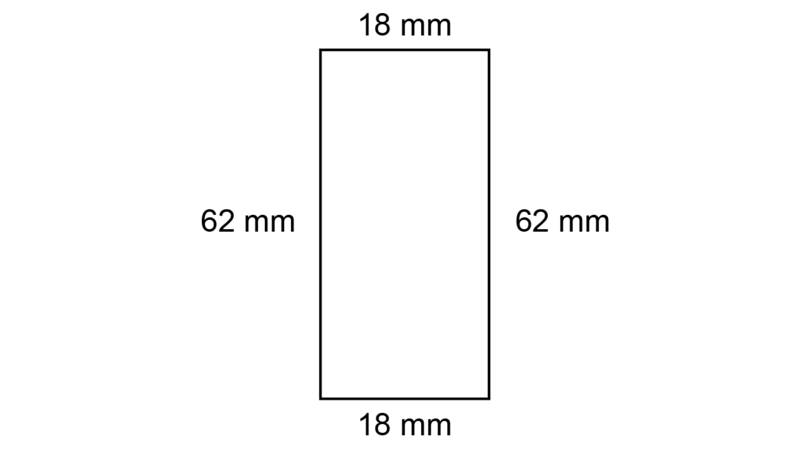
Perimeter=18 mm+62 mm+18 mm+62 mm=160 mm
The name of the polygon: rectangle.
-
Give the name of the irregular polygon shown in the diagram, and find its perimeter.

Perimeter=5 m+7 m+3 m+10 m=25 m
The name of the polygon: trapezium.
-
Give the name of the irregular polygon shown in the diagram, and find its perimeter.

Perimeter=120 mm+80 mm+120 mm+80 mm=400 mm
The name of the polygon: parallelogram.
-
Study the diagram below and calculate the perimeter of this shape by using the given lengths in cm.
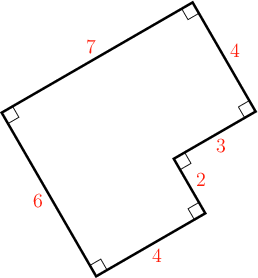
The perimeter of a two-dimensional object is the distance around its edge. The diagram below shows the perimeter in red and blue.
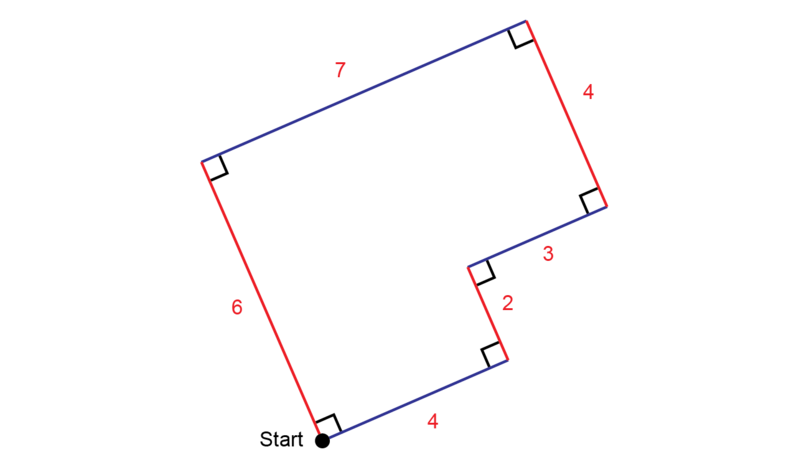
To find the perimeter, we need to add up all six sides of the shape. We can start at any corner we want and go around until we get back where we started. We will start at the corner labelled "Start".
Perimeter =Sum of lengths of sides=6+7+4+3+2+4=26 cmYou do not have to start at the same corner: you can start at any corner and you will get the same total distance. This is because the distance around the shape is the same no matter where you start.
-
Study the diagram below and calculate the perimeter of this object by using the given lengths in m.
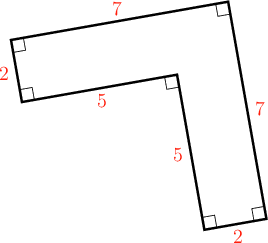
The diagram below shows the perimeter in red and blue.
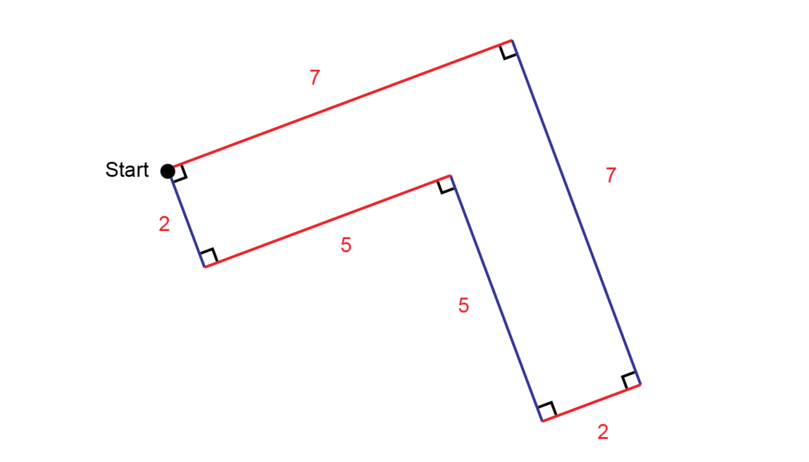
We will start at the corner labelled "Start".
Perimeter=Sum of lengths of sides=7+7+2+5+5+2=28 m -
The diagram below shows a view from above Ms Chidi's house and land. The property has a fence going around the outside. What is the perimeter of the property, if measurements are given in metres?
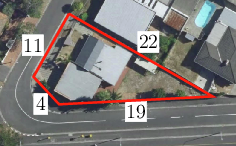
To calculate the perimeter, we add up all the boundary lines that form the perimeter of this property.
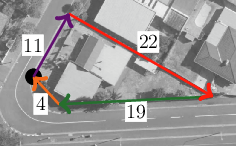
If we start at the black dot shown on the diagram and walk all around the boundary, we get:
Perimeter=11+22+19+4=56 mThe perimeter of the property is 56 m.
Formulae to find the perimeter of a rectangle and a square
It can take long to find the perimeter of a polygon by counting the steps or adding the lengths of the sides. Luckily, there are formulae that we can use for some shapes. The formulae are based on the fact that the perimeter of a shape is the distance around the shape, and that these shapes have some sides that are equal.
Worked example 12.8: Using a formula to find the perimeter of a rectangle
Find the perimeter of the rectangle given below.

-
Step 1: Look at the diagram and take note of the information given. Fill in the missing lengths.
The opposite sides of a rectangle are the same length. Let the longer side be l and the shorter side be b.

-
Step 2: Think of a shorter way than adding all the side lengths.
In a rectangle, you know that the two lengths are equal, and the two breadths are equal. This means you can use a formula to calculate the perimeter of a rectangle.
Perimeter of rectangle=l+b+l+b=2×l+2×b=2l+2b -
Step 3: Fill in the correct values.
Perimeter of rectangle=2l+2b=2×15+2×4 -
Step 4: Do the calculation, using BODMAS to remember the order of operations, so you need to multiply before you add. Write in the correct units at the end of the answer.
Perimeter of rectangle=2l+2b=2×15+2×4=38 cm
For any parallelogram, including a rectangle, the two lengths are the same length, and the two breadths are the same length. This means we can use the formula we reached in the worked example above to calculate the perimeter of a parallelogram:
Perimeter of parallelogram=2l+2bWorked example 12.9: Using a formula to find the perimeter of a square
Find the perimeter of the square given below.
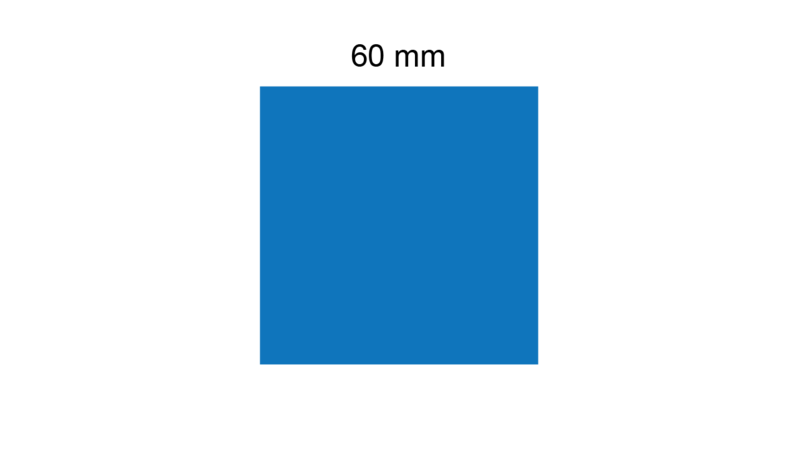
-
Step 1: Look at the diagram and take note of the information given. Fill in the missing lengths. In a square all four sides are equal.
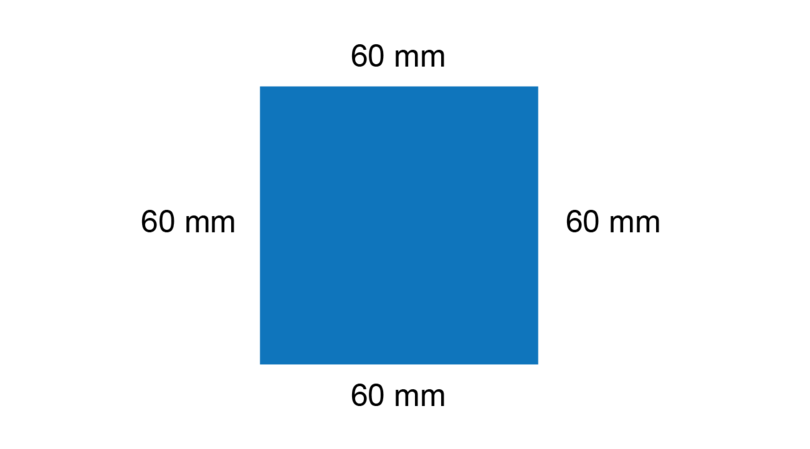
-
Step 2: Think of a shorter way than adding all the side lengths.
All of the sides of a square are the same length. If we let the side be s, then the perimeter is:
Perimeter of square=s+s+s+s=4×s=4s -
Step 3: Fill in the correct values.
Perimeter of square=4s=4×60 -
Step 4: Do the calculation, and remember to add the correct units to the answer.
Perimeter of square=4s=4×60=240 mm
The four sides of a square and of a rhombus are all equal. We can therefore use the following formula to calculate the perimeter of a square and a rhombus:
Perimeter of square and rhombus=4sFormulae to calculate perimeter
The table below lists the formulae for the perimeter of the two-dimensional shapes you will be working with. In a formula, we can use P as a symbol for perimeter.
| Shape | Perimeter formula |
|---|---|
| rectangle | P=2l+2b |
| parallelogram | P=2l+2b |
| square | P=4s |
| rhombus | P=4s |
| triangle | P=side 1+side 2+side 3=s1+s2+s3 |
| trapezium | P=side 1+side 2+side 3+side 4=s1+s2+s3+s4 |
Exercise 12.6: Calculate perimeter using formulae
-
Calculate the perimeter of the rectangle given below.

Perimeter of rectangle=2l+2b=2×50+2×15=100+30=130 mm
-
Calculate the perimeter of the square given below.
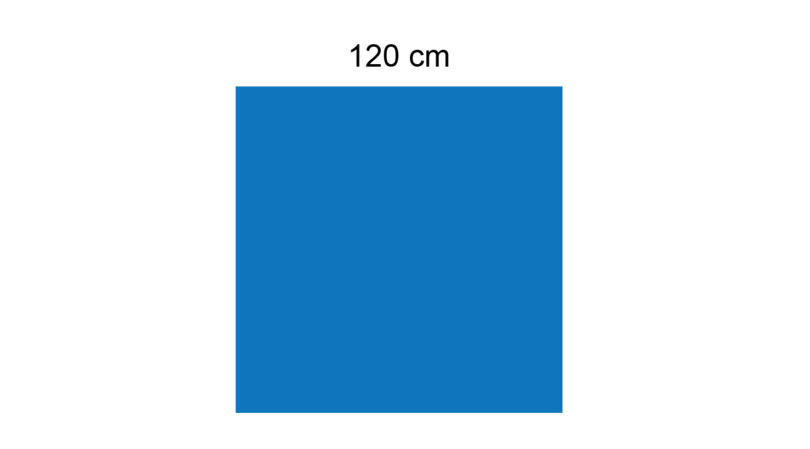
\begin{align} \text{Perimeter of square}& = 4s\\ &= 4 \times \text{120} \\ &= \text{480 cm} \end {align}
-
Calculate the perimeter of the triangle given below.
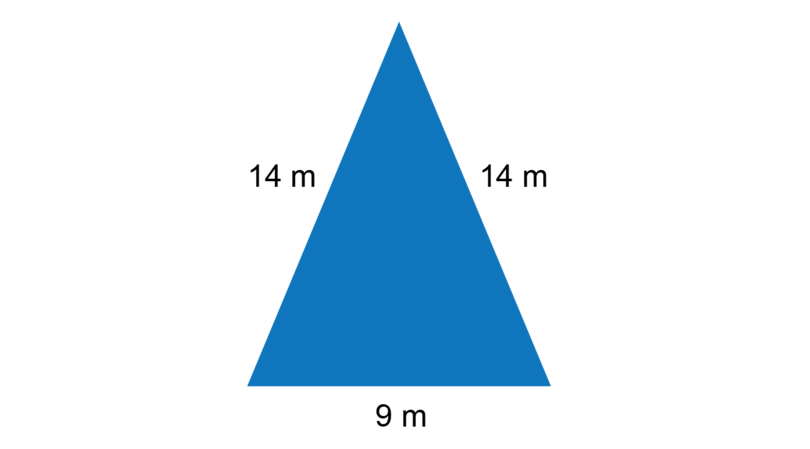
\begin{align} \text{Perimeter of triangle}& = s_1 + s_2 + s_3 \\ &= \text{14} + \text{14} + \text{9} \\ &= \text{37 m} \end {align}
-
Calculate the perimeter of the parallelogram given below.

\begin {align} \text{Perimeter of parallelogram}& = 2l + 2b \\ &= 2 \times \text{90} + 2 \times \text{20} \\ &= \text{180} + \text{40} \\ & = \text{220 mm} \end {align}
-
Calculate the perimeter of the trapezium given below.
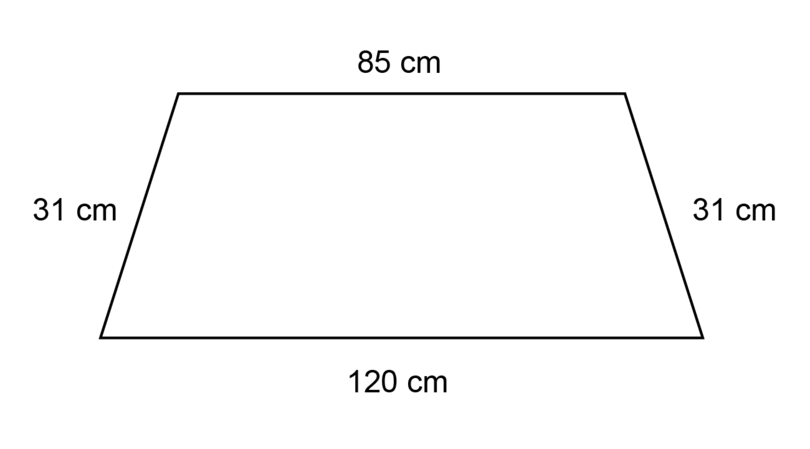
\begin{align} \text{Perimeter of trapezium}& = s_1 + s_2 + s_3 + s_4\\ &= \text{31} + \text{85} + \text{31} + \text{120}\\ &= \text{267 cm} \end {align}
-
Consider the following square. Determine the perimeter of the square.
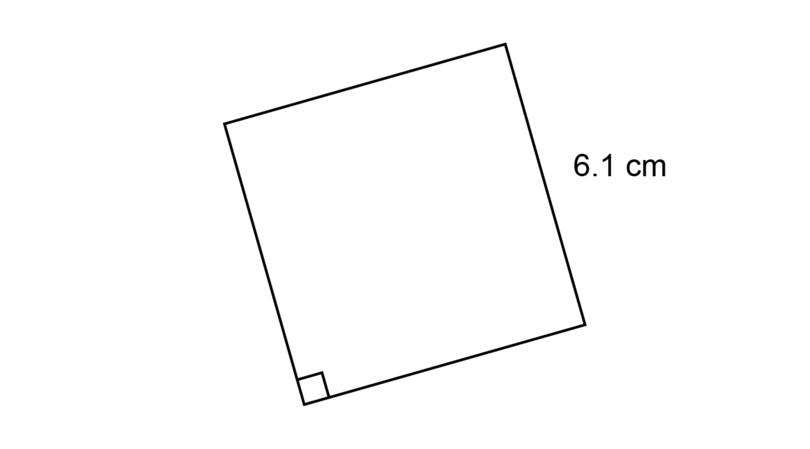
\begin{align} \text{Perimeter of square}& = 4s\\ &= 4 \times \text{6.1} \\ &= \text{24.4 cm} \end {align}
-
Consider the following rectangle. Determine the perimeter of the rectangle.
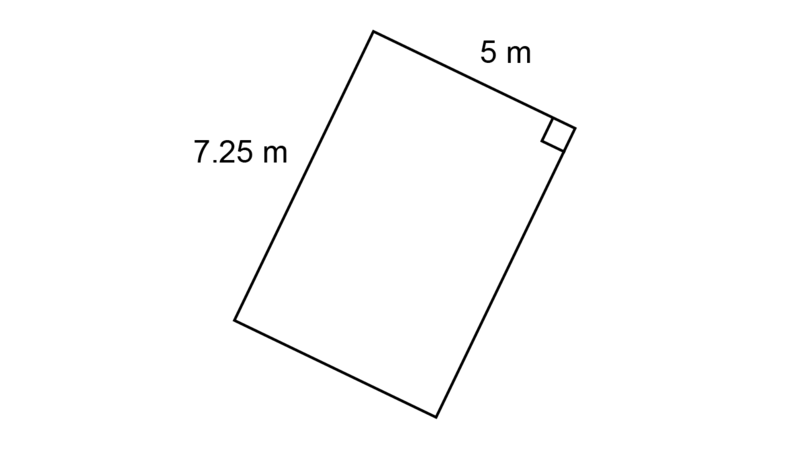
Perimeter of rectangle=2l+2b=2×7.25+2×5=14.5+10 =24.5 m
-
Calculate the perimeter of a triangle with the following side lengths: 14m;13m and12m.
In your calculation, Remember to find the lowest common denominator of the fractions before you add them.
\begin {align} \text{Perimeter of triangle}& = s_1 + s_2 + s_3 \\ &= \tfrac {1}{4} + \tfrac {1}{3} + \tfrac {1}{2} \\ &= \tfrac {3}{12} + \tfrac {4}{12} + \tfrac {6}{12} \\ &= \tfrac {13}{12} \\ &= 1 \tfrac {1}{12} \text{m} \end {align} -
Calculate the perimeter of a rhombus with side length 38 km.
\begin{align} \text{Perimeter of rhombus}& = 4s\\ &= 4 \times \tfrac {3}{8} \\ &= \tfrac {4 \times 3}{1 \times 8}\\ &= \tfrac {12}{8} \\ &= \tfrac {3}{2} \\ &= 1 \tfrac {1}{2} \text{km} \end {align}
-
Calculate the perimeter of the trapezium given below.
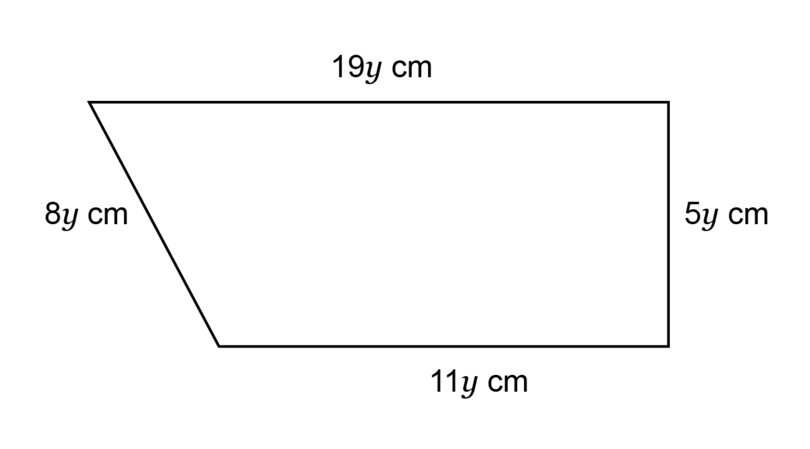
\begin{align} \text{Perimeter of trapezium}& = s_1 + s_2 + s_3 + s_4 \\ &= 19y + 5y + 11y + 8y \\ &= 43y \text{ cm} \end {align}
-
The diagram below shows a notebook. What is the perimeter of the notebook if all the measurements are given in centimetres?

The notebook is in the shape of a rectangle.
\begin {align} \text{Perimeter of rectangle} & = 2l + 2b \\ &= 2 \times 25 + 2 \times 15 \\ &= \text{50} + \text{30} \\ &= \text{80 cm} \end {align}The perimeter of the notebook is 80 cm.
Using a formula to find the circumference of a circle
We know that the perimeter of a circle is called its circumference. The circumference of a circle is a curve, so we cannot measure it with a ruler. We can use a formula to calculate the circumference of a circle. There are two formulae that we can use, which both mean the same thing:
C=π×d or C=2×π×rIn these formulae, C stands for circumference, d stands for the diameter of the circle, and r stands for the radius of the circle. We will mostly use the first formula with diameter, but you can use the radius formula if you prefer.
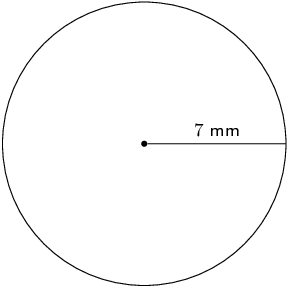
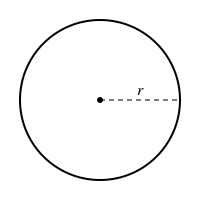
radius The radius of a circle is the distance from the centre to the circumference.
diameter The diameter of a circle is the length of a line passing through the centre of the circle, and touching the circumference at both ends.
The diameter of a circle is double the radius, so d=2×r.
You will see that the symbol π is used in both formulae. This name of this symbol is "pi". π is the ratio of the circumference of a circle to its diameter.
π=CdThe value of π is the same for all circles, large or small. We can see this by doing a quick experiment. Draw a circle with a compass. Place a piece of string along the circumference, and then measure the length of the string using a ruler. Next, use the ruler to measure the diameter of the circle. If we divide the circumference by the diameter, we will get a value that is approximately 3.14. If we do the same thing, but with a larger or a smaller circle, we will get the same value, every time!
π π (pi) is the ratio of the circumference of a circle to its diameter. The values we use for π are 3.14 and 227.
Look at the value of π that your calculator gives you, and compare this to the value of 227. Are they the same number?
π is an unending number approximately equal to 227 or 3.14. Here are the first 200 digits of π:
3.14159265358979323846264338327950288419716939937510582097494459230781640628620899862803482534211706798214808651328230664709384460955058223172535940812848111745028410270193852110555964462294895493038196...Scientists using powerful computers have calculated the value of π to over 13 trillion decimal places. But they will never be able to reach the end of this number, since the end does not exist.
When we do calculations with π, we cannot use the exact value of π. Instead, we use an approximation of the value of π. One commonly used value is 3.14, which is π rounded to two decimal places. Another approximation is 227. If we divide 22 by 7 on a calculator, we get 3.142857143. This is very close to π to two decimal places, but it is a slightly better approximation.
Worked example 12.10: Calculating circumference using diameter
The circle below has a diameter of 8 m. What is the circumference of this circle?

-
Step 1: Write down the formula for the circumference of a circle:
C=π×d -
Step 2: The question tells us that the diameter of the circle is 8 m. Substitute 8 for the d in the formula to find the circumference of the circle.
We will use 3.14 for π.
C=π×d=3.14×8 -
Step 3: Do the calculation and remember to show the correct units with the answer.
C=π×d=3.14×8=25.12 mThe circumference of the circle is 25.12 m.
If you have a π button on your calculator, you may use it to work out the answer. But if the question gives you a value to use, such as 3.14, use that value.
Worked example 12.11: Calculating circumference using radius
The circle below has a radius of 2 cm. What is the circumference of the circle? Use 3.14 for π.

-
Step 1: Write down the formula for the circumference of a circle.
C=π×dOr you can use C=π×2r
-
Step 2: The question tells us that the radius of the circle is 2 cm. So the diameter is 4 cm. Substitute 4 for for the d in the formula. Also substitute 3.14 for π.
C=π×d=3.14×4As you can see, if you used C=π×2r, you would also get 3.14×4.
-
Step 3: Calculate the circumference of the circle. Remember to show the correct units in your answer.
C=π×d=3.14×4=12.56 cmThe circumference of the circle is 12.56 cm.
Exercise 12.7: Calculate the circumference of a circle
Use 3.14 for π in all your calculations.
-
The circle below has a diameter of 9 cm. What is the circumference of the circle?
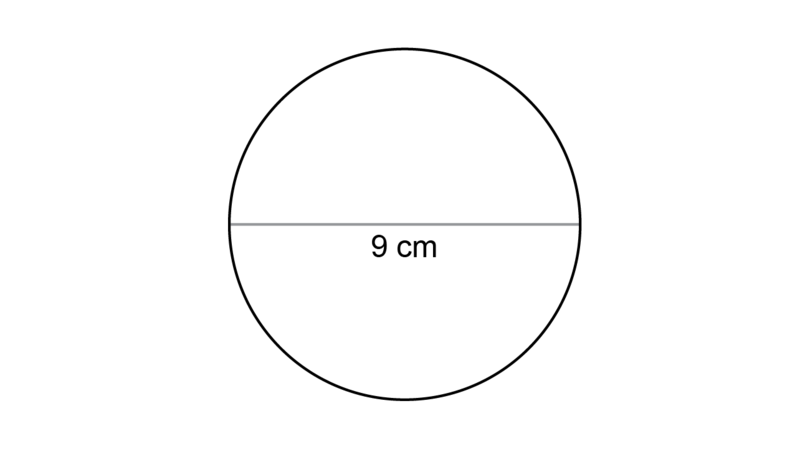
C=π×d=3.14×9=28.26 cm
-
The circle below has a radius of 5 cm. What is the circumference of the circle?

If the radius is 5 cm, then the diameter is 10 cm.
C=π×d=3.14×10=31.4 cm -
If a circle has a diameter of 11 m, what is the circumference of the circle?
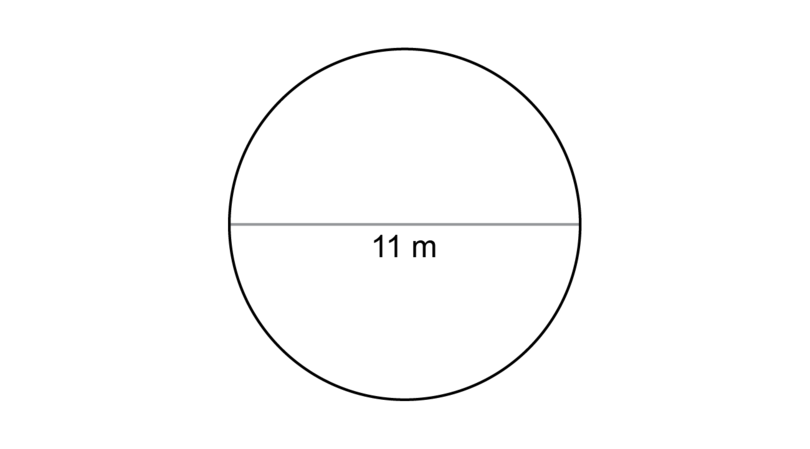
C=π×d=3.14×11=34.54 m
-
If a circle has a radius of 10 m, what is the circumference of the circle?

If the radius is 10 cm, then the diameter is 20 cm.
C=π×d=3.14×20=62.8 m -
What is the circumference of a circle that has a diameter of 40 mm?
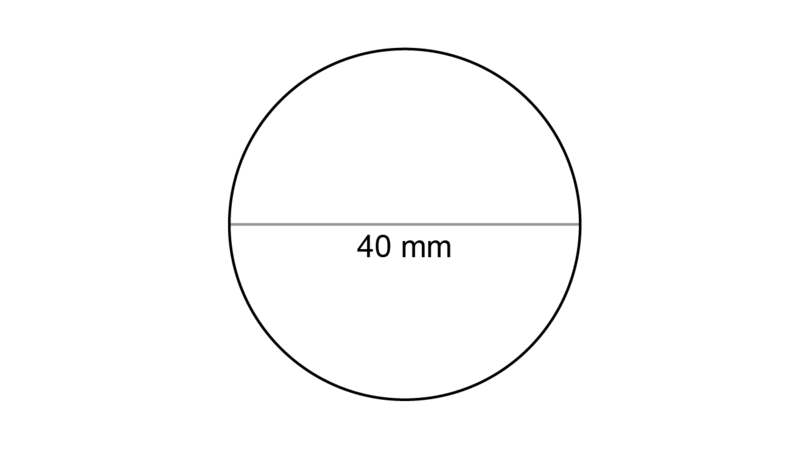
C=π×d=3.14×40=125.6 mm
-
What is the circumference of a circle that has a radius of 50 mm?

If radius is 50 mm, then diameter is 100 mm.
C=π×d=3.14×100=314 mm
12.3 Area of two-dimensional shapes
Area is the amount of flat space taken up by a shape. So we can think of area as the surface of a shape that you can cover or paint. For example, the space inside the white line painted around a football field is the area of the football field.
area Area is the amount of flat space taken up by a shape.
Units of measurement for area
We measure the area of a two-dimensional shape by counting or working out how many unit squares will fit into the space. Examples of units of measurement for area are mm2, cm2, m2, or even km2.
-
A square millimetre (mm2) is a tiny square with sides each one millimetre long. It is about the size of a flattened breadcrumb.
-
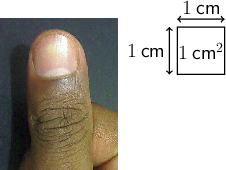
A square centimetre (cm2) is the size of a square with sides that are one centimetre long. It is about the size of your thumbnail.
-
A square metre (m2) is the size of a square with sides each one metre long. It is about the space on the floor that you need to sit cross-legged.
-
A square kilometre (km2) is the space inside a square with sides that are one kilometre long. It is a very big unit for measuring area. You can fit about ten football fields into one square kilometre.
Determine area by counting squares on grid
If a diagram is drawn on a grid, you can simply count the squares to find the area of the shape.
Each small square on the grid can represent a square in real life.
-
One small square on the grid can represent an area of 1 m by 1 m: 1 m×1 m=1 m2.
-
One small square on the grid can represent an area of 1 cm by 1 cm: 1 cm×1 cm=1 cm2.
Worked example 12.12: Counting squares to find the area of a rectangle
Consider the diagram of a rectangle below. Use the grid to determine the area of the rectangle. Each small square on the grid represents a square of 1 km by 1 km.
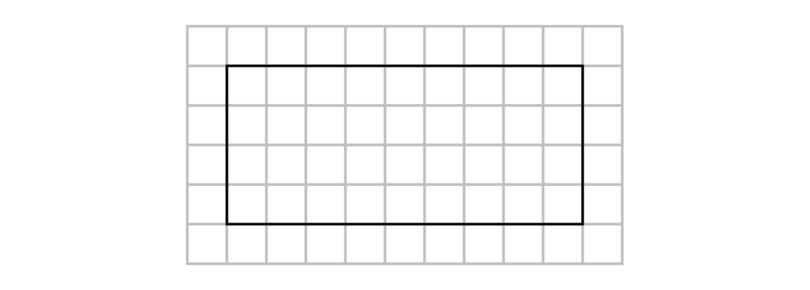
-
Step 1: Remember that area is the amount of flat space taken up by a shape.
To determine area, you count the number of squares on the grid that are covered by the given shape.
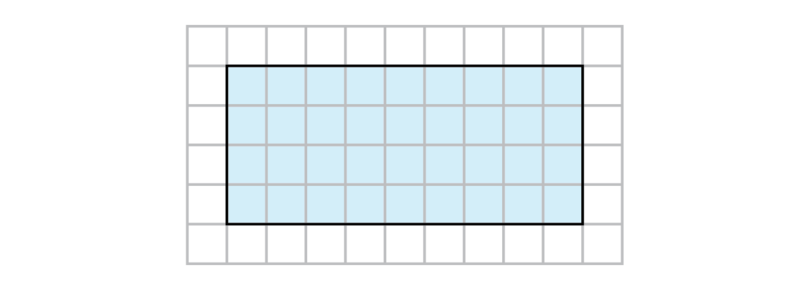
There are 36 squares inside the rectangle.
-
Step 2: Give the answer in the correct square units.
The area of the rectangle is 36 square kilometres = 36 km2.
Worked example 12.13: Counting squares to find the area of a square
Determine the area of the square shown below by counting the number of smaller squares on the grid that are covered by the square. Each square on the grid represents a square of 1 cm by 1 cm.
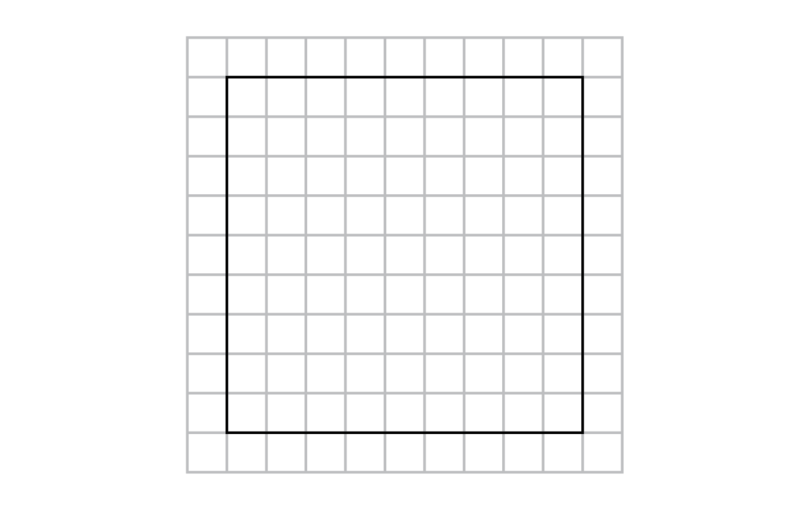
-
Step 1: Count the number of squares on the grid that are covered by the given square.
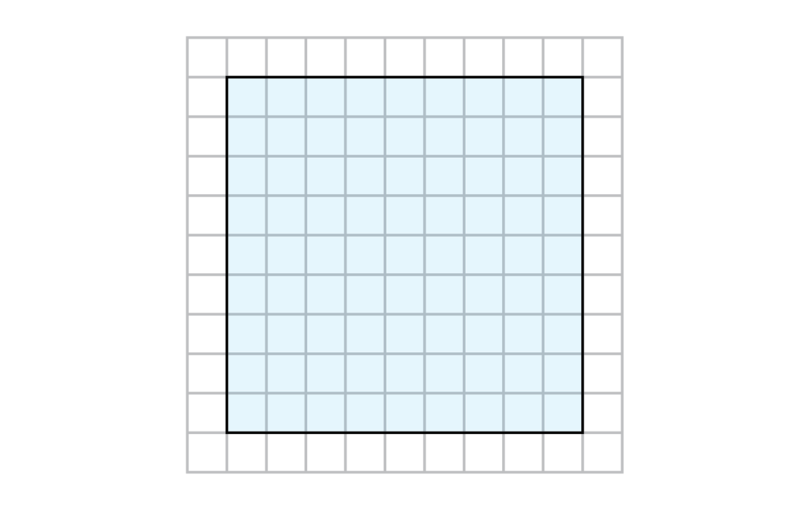
-
Step 2: Write down the number of squares.
There are 81 smaller squares covered by the given square.
-
Step 3: Give the answer in the correct square units.
The area of the given square is 81 square centimetres = 81 cm2.
Exercise 12.8: Counting squares to determine area
-
Consider the diagram of a rectangle below. Determine the area of the rectangle by counting the squares on the grid. Each small square represents 1 m by 1 m.

Count the number of squares covered by the rectangle.
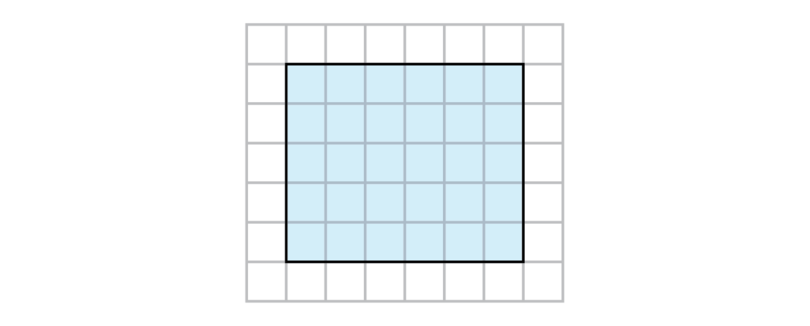
The rectangle takes up 30 squares, so there are 30 square metres covered by the rectangle.
Area of rectangle = 30 m2
-
Determine the area of the square shown below by counting the smaller squares on the grid. Each small square represents 1 cm by 1 cm.
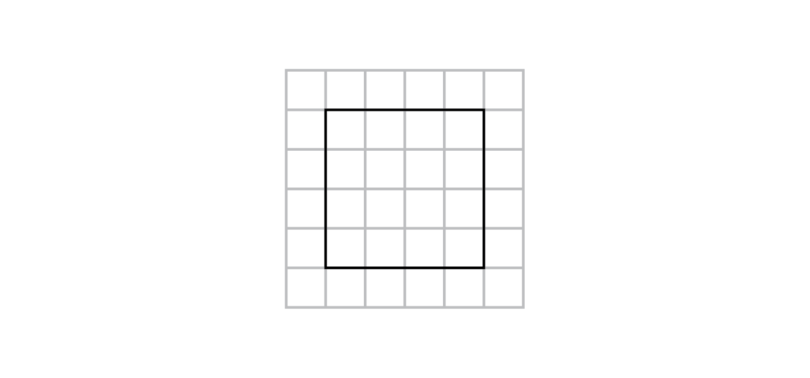
Count the number of squares covered by the given square.
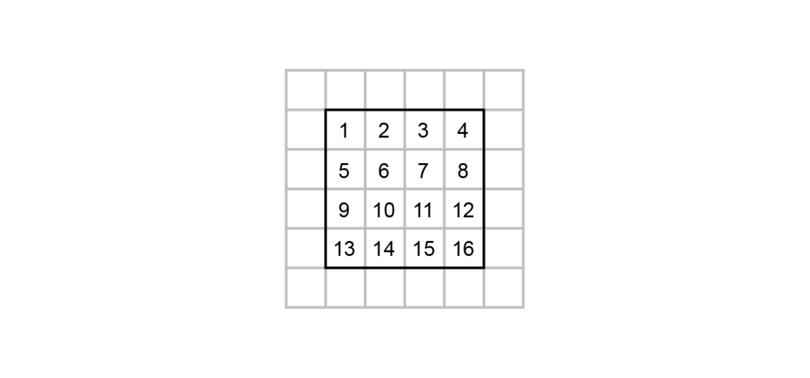
The square takes up 16 smaller squares, so there are 16 square centimetres covered by the square.
Area of square = 16 cm2
-
Determine the area of the polygon provided. Each small square represents 1 cm by 1 cm.
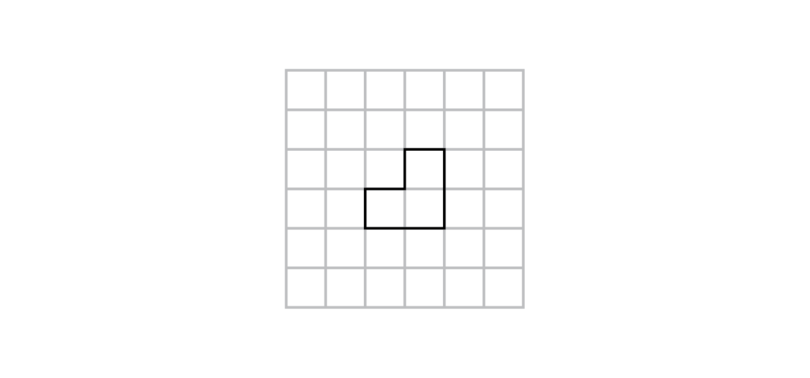
Count the number of squares covered by the polygon.
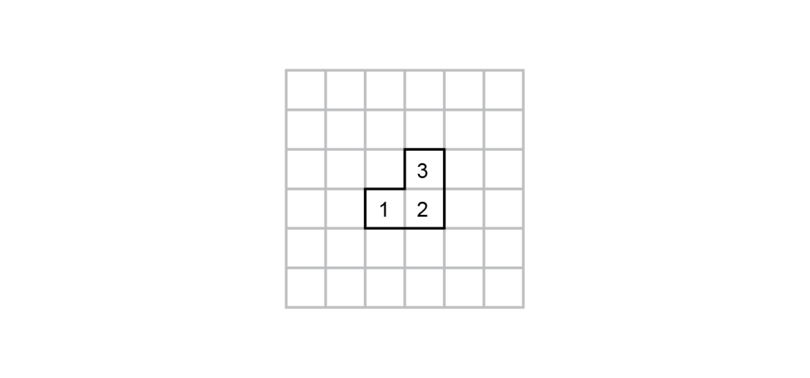
The polygon takes up 3 squares, so there are 3 square centimetres covered by the polygon.
Area of polygon = 3 cm2
-
Determine the area of the polygon. Each square represents 1 m by 1 m.
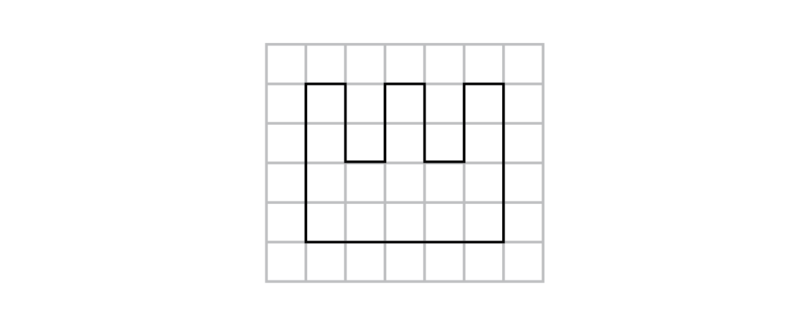
Count the number of squares covered by the polygon.
The polygon takes up 16 squares, so there are 16 square metres covered by the polygon.
Area of polygon = 16 m2
-
Determine the area of the triangle shown below. Each small square represents 1 cm by 1 cm.
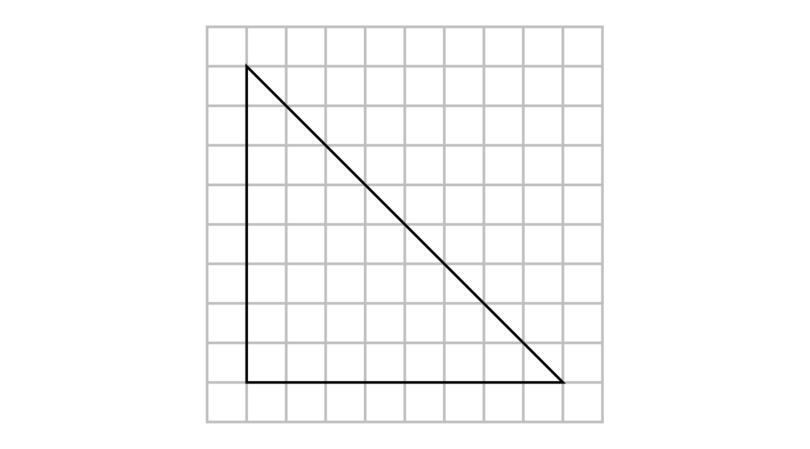
Count the number of smaller squares covered by the triangle.
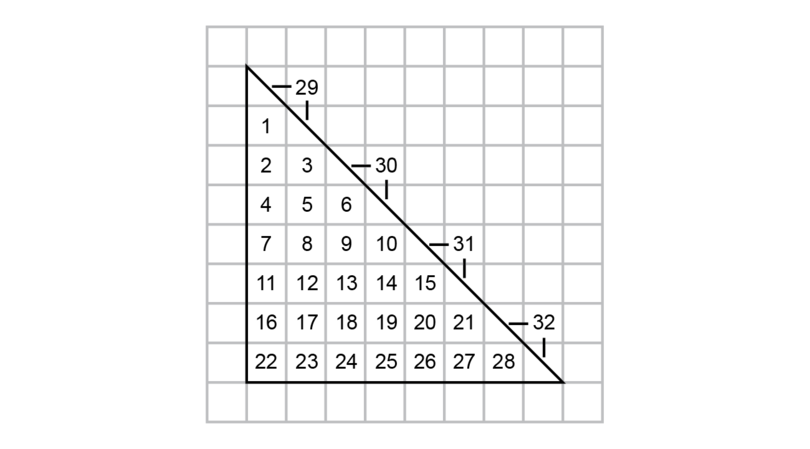
The triangle takes up 28 full squares and 8 half squares. 8 half squares = 4 squares, and 28 + 4 = 32, so there are 32 square centimetres covered by the triangle.
Area of triangle = 32 cm2
Using formulae to calculate area
If a shape is not drawn on a grid, you cannot find the area of the shape by counting the squares, but there are formulae that you can use to calculate area.
You can check that the formulae are correct by comparing the answers when you use the two different methods. The method of counting the squares should give you the same answer as when you use a formula to calculate the area of a shape.
In the two worked examples below, you will first count the squares and then use a formula to find the area. In each case you will see that the two answers are the same.
Worked example 12.14: Comparing methods of calculating area of a rectangle
Find the area of the rectangle provided by counting squares. Each square on the grid represents a square of 1 km by 1 km. Then work out a formula for calculating the area of a rectangle, and check the answer you get by comparing it to your first answer.

-
Step 1: Remember that area is the amount of flat space taken up by a shape. Count the number of squares on the grid that are covered by the rectangle.
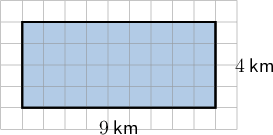
The rectangle consists of 9 columns of smaller squares. Each column has 4 smaller squares. So there are 9 × 4 = 36 squares inside the rectangle.
Area of rectangle = 36 km2
-
Step 2: Use your calculation above to work out a formula to calculate the area of a rectangle.
You can see that 9 km is the length of the rectangle, and 4 km is the breadth. To get the area, you multiplied 9 by 4, so what you did was multiply the length by the breadth. Then you wrote in the square units, which this time is km2.
So the formula for the area of a rectangle is length × breadth.
-
Step 3: Use the formula to calculate the area.
Area of rectangle=length×breadth=l×b=9 km×4 km=36 km2 -
Step 4: Compare this answer with your first answer.
Both methods give the same answer: A = 36 km2.
Worked example 12.15: Comparing methods of calculating area of a square
Find the area of the square provided by counting squares. Each square on the grid represents a square of 1 cm by 1 cm. Then work out a formula for calculating the area of a square, and check the answer you get by comparing it to your first answer.
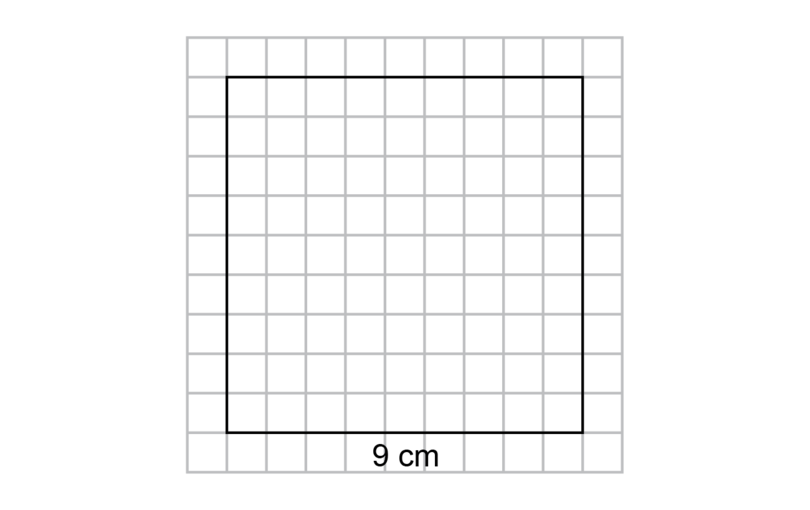
-
Step 1: Count the number of squares on the grid that are covered by the square.
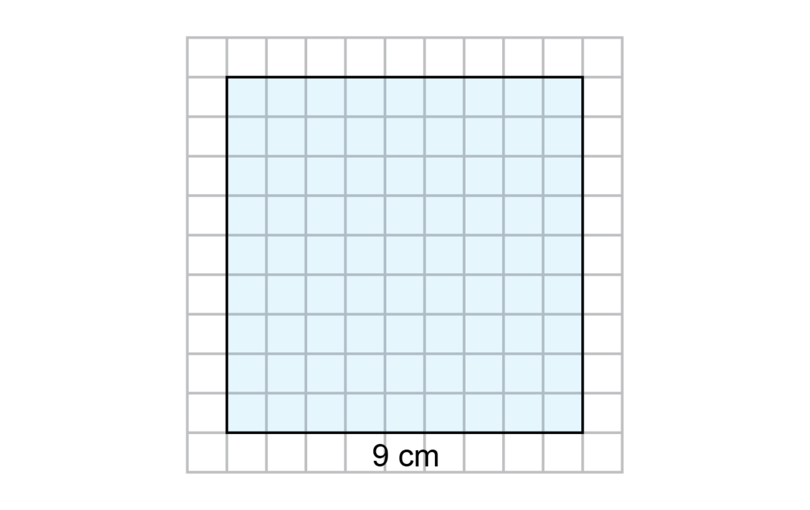
The square consists of 9 rows of squares and 9 columns of squares. So there are 9 × 9 = 81 squares inside the square.
Area of square = 81 cm2
-
Step 2: Use your calculation to work out a formula to calculate the area of a square.
In a square, all four sides are the same length. To get the area, you multiplied 9 by 9, so what you did was multiply the length by the length (or we can say the side by the side). So the area is just the product of the two (equal) sides. Then you wrote in the square units, which this time is cm2.
So the formula for the area of a square is side × side.
-
Step 3: Use the formula to calculate the area.
Area of square=side×side=9 cm×9 cm=81 cm2 -
Step 4: Compare this answer with your first answer.
Both methods give the same answer: A = 81 cm2.
Formulae for the area of a two-dimensional shape
It will help you if you memorise the formulae for area, so that you can use them easily when you need to. Understanding where the formulae come from will help you to memorise them.
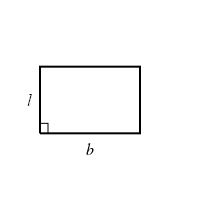
- Rectangle: Area of a rectangle = length × breadth.
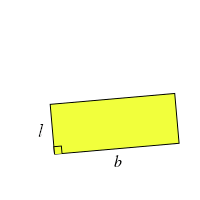
-
Square: Area of a square =s×s=s2
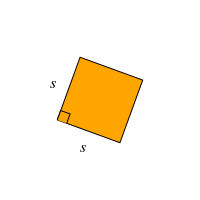
-
Triangle: Area of a triangle =b×h2.
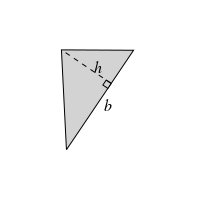
The base (b) and the height (h) of a triangle are labelled in the diagram above. It is very important with a triangle that the base and the height are perpendicular to each other, or you will not get the correct answer.
-
Parallelogram: Area of a parallelogram =b×h.
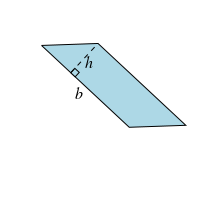
It is also important that the base (b) and the height (h) of a rectangle are perpendicular to each other.
-
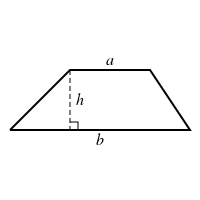
Trapezium: Area of a trapezium =h2(a+b).
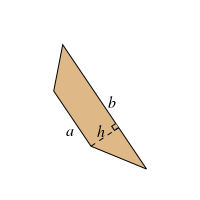
The two parallel sides of the trapezium (a and b) must be perpendicular to the height (h) of the trapezium.
-
Circle: Area of a circle =π×r2.
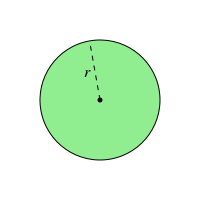
Remember that r is the radius of the circle.
Summary of the area formulae
In the formulae, we can use A to stand for area.
| Shape | Area formula |
|---|---|
| rectangle | A=l×b |
| square | A=s×s=s2 |
| triangle | A=b×h2 |
| parallelogram | A=b×h |
| trapezium | A=h2(a+b) |
| circle | A=π×r2 |
Exercise 12.9: Identify the correct area formula
-
For each of the shapes shown below, identify the correct formula for the area of the shape.
Shape A Shape B 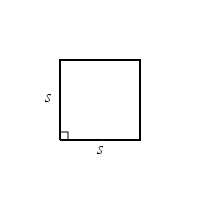
Choice Area formula 1 A=π×r2 2 A=s2 3 A=b×h2 4 A=l×b 5 A=h2(a+b) 6 A=l×b Shape A is a square, so the formula is 2: A=s2
Shape B is a circle, so the formula is 1: A=π×r2
-
Identify the correct formula for the area of the given shape.
Shape A Shape B 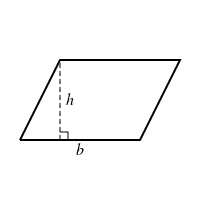
Choice Area Formula 1 A=π×r2 2 A=s2 3 A=b×h2 4 A=b×h 5 A=h2(a+b) 6 A=l×b Shape A is a parallelogram, so the formula is 4: A=b×h
Shape B is a trapezium, so the formula is 5: A=h2(a+b)
-
Which is the correct formula for the area of the shapes provided?
Shape A Shape B 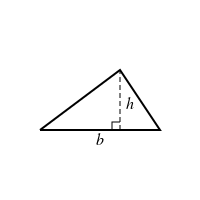
Choice Area Formula 1 A=π×r2 2 A=b2 3 A=b×h2 4 A=b×h 5 A=h2(a+b) 6 A=l×b Shape A is a triangle, so the formula is 3: A=b×h2
Shape B is a rectangle, so the formula is 6: A=l×b
Using formulae to calculate area
It saves lots of time if you know the correct formula to use when you have to calculate the area of a shape. Then you simply write down the correct formula, substitute the given values into the formula, and do the calculation. Always remember to show the correct units with the final answer.
Worked example 12.16: Finding area of a rectangle using a formula
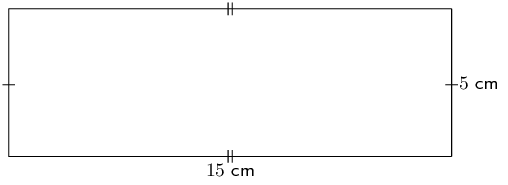
Find the area of the rectangle shown below.
-
Step 1: Write down the correct formula for the area of a rectangle.
A=l×b -
Step 2: Substitute the correct values into the formula.
A=l×b=15 cm×5 cm -
Step 3: Do the calculation and remember to show the correct units in your answer.
A=l×b=15 cm×5 cm=75 cm2
Worked example 12.17: Finding area of a square using a formula
Find the area of the square shown below.
-
Step 1: Write down the correct formula for the area of a square.
A=s×s -
Step 2: Substitute the correct values into the formula.
A=s×s=7 m×7 m -
Step 3: Do the calculation and remember to show the correct units in your answer.
A=s×s=7 m×7 m=49 m2
When we do calculations we normally do not show all the units while we work. We only show the correct unit when we give the final answer. The units are shown in the examples above to demonstrate that 1 cm×1 cm=1 cm2, and 1 m×1 m=1 m2. The same applies to all units of measurement that are used.
Worked example 12.18: Finding area of a triangle using a formula
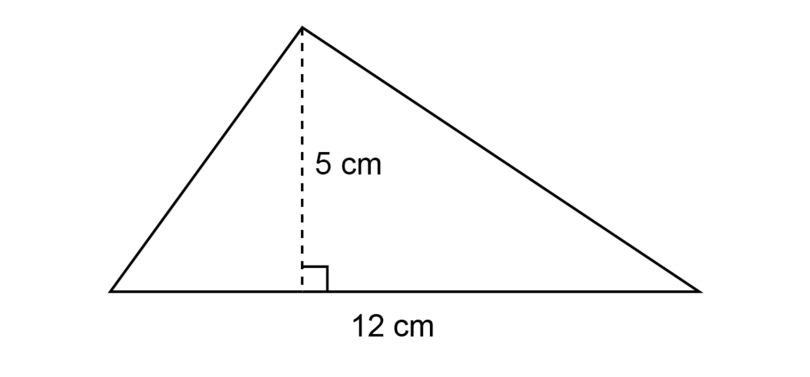
Find the area of the triangle shown below.
-
Step 1: Write down the correct formula for the area of a triangle.
A=b×h2 -
Step 2: Substitute the correct values into the formula.
A=b×h2A=12×52 -
Step 3: Do the calculation and remember to show the correct units in your answer.
A=b×h2A=12×52A=602A=30 cm2
Worked example 12.19: Finding area of a parallelogram using a formula
Find the area of this parallelogram.

-
Step 1: Write down the correct formula for the area of a parallelogram.
A=b×h -
Step 2: Substitute the correct values into the formula.
A=b×h=20×14 -
Step 3: Do the calculation and remember to show the correct units in your answer.
A=b×h=20×14=280 cm2
Worked example 12.20: Finding area of a trapezium using a formula
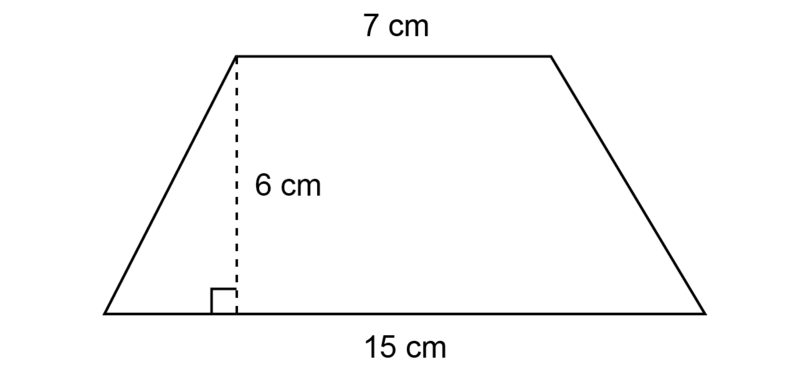
Find the area of this trapezium.
-
Step 1: Write down the correct formula for the area of a trapezium.
A=h2(a+b) -
Step 2: Substitute the correct values into the formula.
A=h2(a+b)=62(7+15) -
Step 3: Do the calculation and remember to show the correct units in your answer.
A=h2(a+b)=62(7+15)=3×22=66 cm2
Worked example 12.21: Finding area of a circle using a formula
Find the area of the circle shown below.
-
Step 1: Write down the correct formula for the area of a circle.
A=π×r2 -
Step 2: Substitute the correct values into the formula. Use 3.14 for π.
A=π×r2=3.14×72 -
Step 3: Do the calculation and remember to show the correct units in your answer.
A=π×r2=3.14×72=3.14×49=153.86 mm2
Exercise 12.10: Use formulae to calculate area
-
Calculate the area of PQRS.

A=l×b=30×20=600 mm2
-
Find the area of the rectangle.

A=l×b=5×7.25=36.25 m2
-
Determine the area of the square.
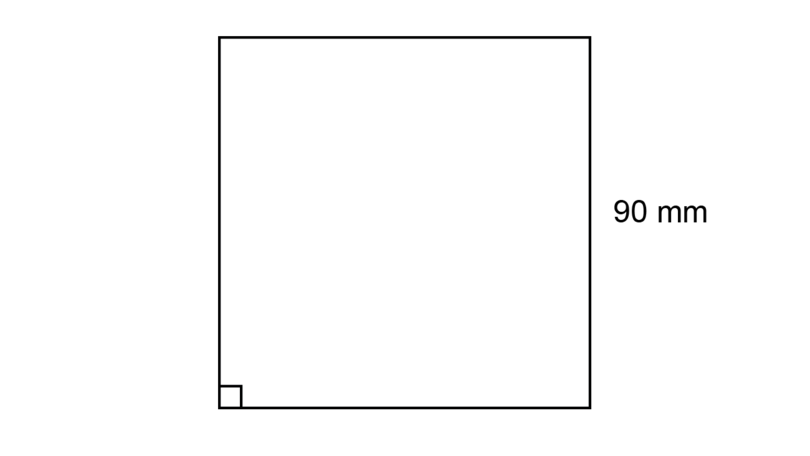
A=s×s=s2=902=8,100 mm2
-
Determine the area of the square.
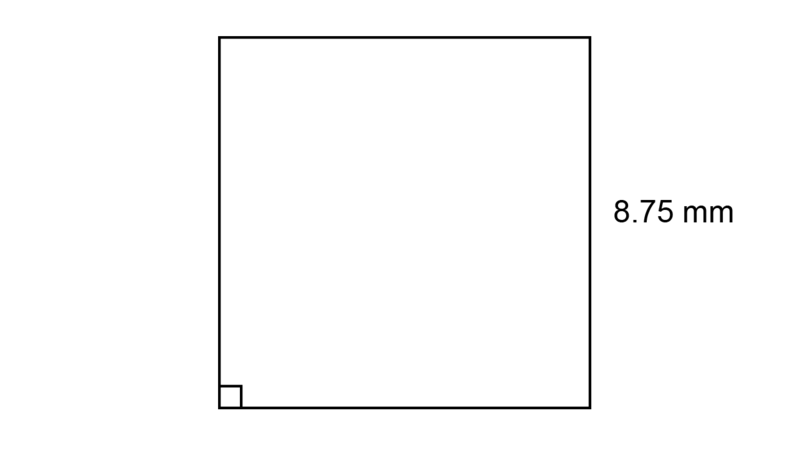
A=s2=8.752=76.5625 mm2
-
Calculate the area of the triangle shown below.
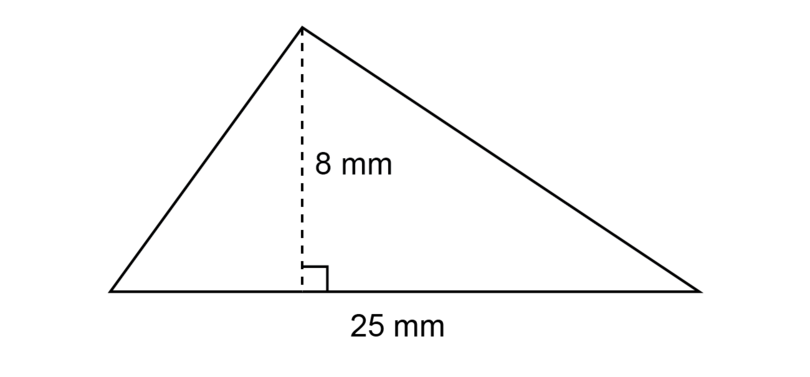
A=b×h2=25×82=2002=100 mm2
-
Calculate the area of the triangle shown below.
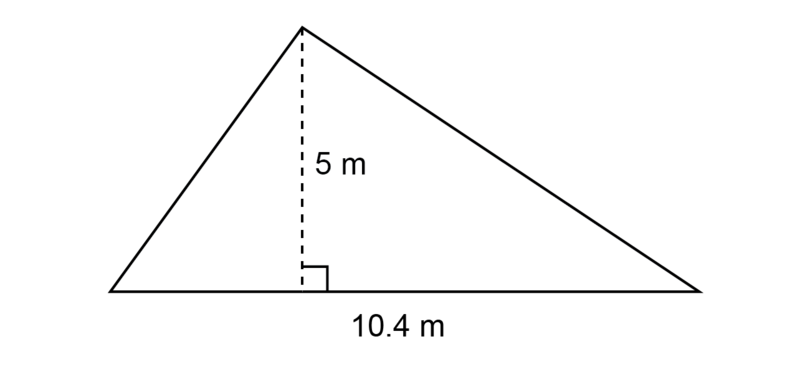
A=b×h2=10.4×52=522=26 m2
-
Find the area of the parallelogram shown below.

A=b×h=40×18=720 m2
-
Determine the area of the parallelogram shown below.

A=b×h=37.5×20=750 mm2
-
Find the area of the trapezium shown below.
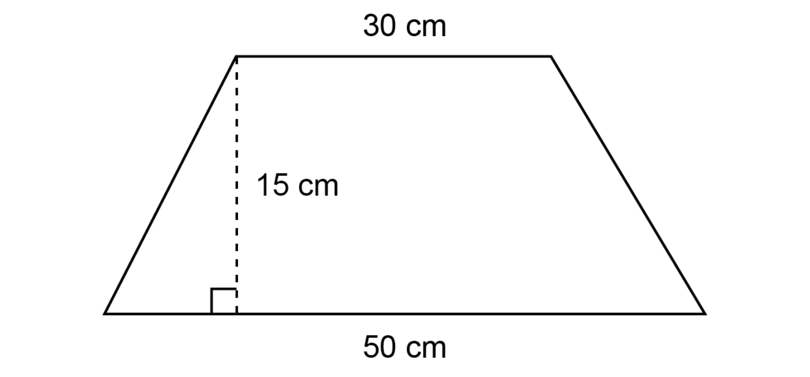
A=h2(a+b)=152(30+50)=7.5×80=600 cm2
-
Find the area of the trapezium shown below.
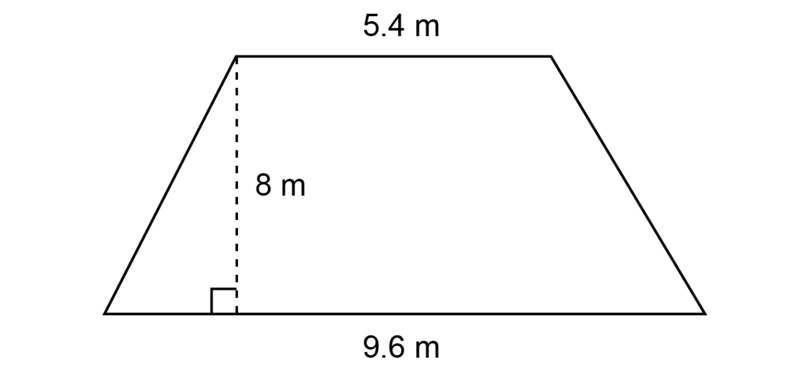
A=h2(a+b)=82(9.6+5.4)=4×15=60 m2
-
The circle below has a radius of 4 m. What is the area of the circle? Use 3.14 for π.
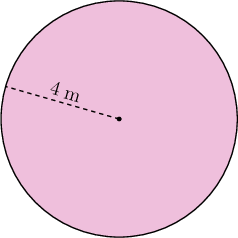
A=π×r2=3.14×42=3.14×16=50.24 m2
-
The circle below has a diameter of 6 km. What is the area of the circle? Use 3.14 for π.
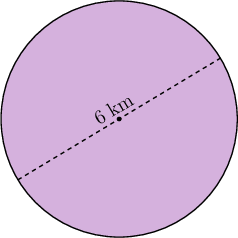
The diameter of a circle is always double the radius, so d=2r. So if the diameter is 6 km, the radius is 3 km.
A=π×r2=3.14×32=3.14×9=50.24 km2 -
If a circle has a radius of 10 mm, what is its area? Use 3.14 for π in your calculation, and round your answer to two decimal places.
A=π×r2=3.14×102=314 mm2
-
If a circle has a diameter of 40 cm, what is its area? Use 3.14 for π, and round your answer to two decimal places.
If d = 40 cm, then r = 20cm.
A=π×r2=3.14×202=3.14×400=1,256 cm2
Area of irregular shapes
Sometimes we have to calculate the area of an irregular shape. Remember that in an irregular polygon, all the sides are not the same length and all the angles are not equal.
Often the irregular shape you are given to work with will be made up of two or more regular shapes. To calculate the area, you divide the irregular shape into simpler shapes, usually squares or rectangles, that have familiar area formulae.
Worked example 12.22: Finding area of irregular shape by counting squares
Determine the area of the given shape. Each square represents a 1 cm×1 cm area.

-
Step 1: See what shapes you can break the shape into.
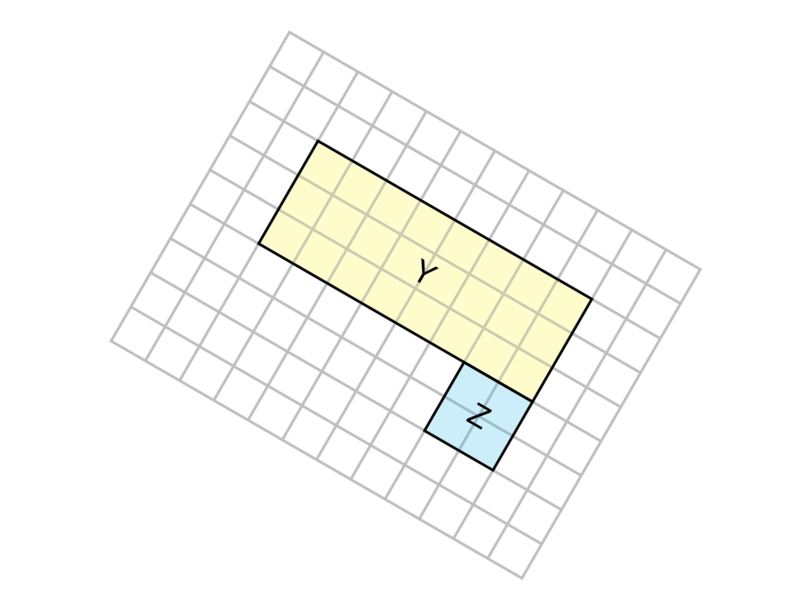
The shape can be divided into the yellow rectangle and the blue square. We will label the yellow rectangle Y, and the blue square Z.
-
Step 2: Calculate the area of the rectangle, Y. Use the formula for the area of a rectangle.
AreaY=length×breadth=8×3=24 cm2 -
Step 3: Calculate the area of the square, Z. Use the formula for the area of a square.
AreaZ=side×side=2×2=4 cm2 -
Step 4: Add the area of the square and the area of the rectangle together to get the total area of the shape. Remember to show the correct units in your answer.
AreaTotal=AreaY+AreaZ=28 cm2Therefore, the area of the shape is 28 cm2.
Worked example 12.23: Finding area of irregular shape by counting squares
Calculate the area of the shape below, using the given lengths in cm.
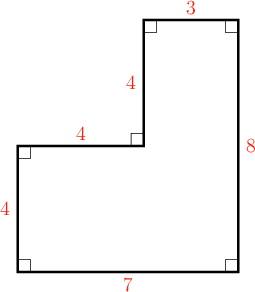
-
Step 1: Break the shape into familiar, regular polygons.
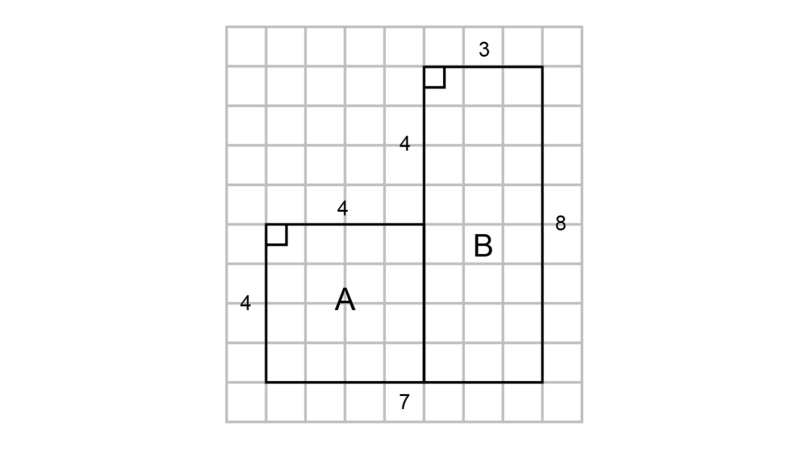
The shape can be divided into a rectangle, labelled B, and a square, labelled A.
-
Step 2: Find the area of the square labelled A.
AreaA=side×side=4×4=16 cm2 -
Step 3: Find the area of the rectangle labelled B.
AreaB=length×breadth=8×3=24 cm2 -
Step 4: Find the sum of the two areas.
AreaTotal=AreaA+AreaB=16 cm2+24 cm2=40 cm2
Exercise 12.11: Calculate the area of irregular shapes
-
Calculate the area of the shape provided. The given lengths are in cm.

Break the shape into two rectangles to find the area. Label the rectangles A and B as shown below.
AreaA=l×b=6×4=24 cm2 AreaB=l×b=4×3=12 cm2AreaTotal=AreaA+AreaB=36 cm2
AreaB=l×b=4×3=12 cm2AreaTotal=AreaA+AreaB=36 cm2 -
Calculate the area of this irregular polygon, using the given lengths in m.

AreaA=l×b=7×2=14 m2 AreaB=l×b=5×2=10 m2AreaTotal=AreaA+AreaB=24 m2
AreaB=l×b=5×2=10 m2AreaTotal=AreaA+AreaB=24 m2 -
In the diagram below, one of the lengths of the sides has been replaced by z. The other five sides have the lengths shown in the figure. All of the angles in the shape are right angles, as labelled.
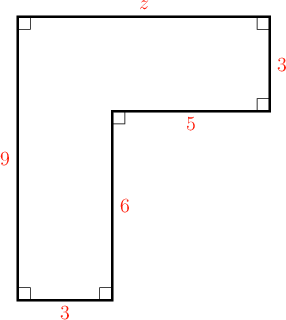
Determine the value of z. Then find the area for this figure. All lengths in the figure have dimensions in mm.
Start by finding z. The sides opposite each other are parallel to each other, because all the angles are right angles. So you can use the sides parallel to z, which are 3 mm and 5 mm long.
The side labelled z has the same length as the two opposite sides added together. So we can write this equation to find z:
z=5+3=8The length of z is 8 mm.
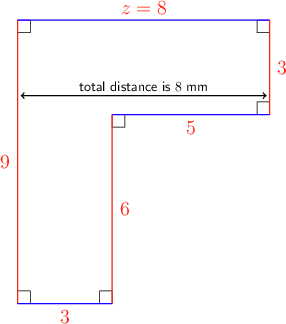
Now we can break the shape into two rectangles to find the area.
AreaA=l×b=9×3=27 mm2AreaB=l×b=3×5=15 mm2AreaTotal=AreaA+AreaB=42 mm2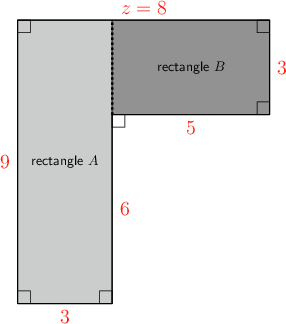
-
In the shape below, one of the lengths of the sides has been replaced by z. The other five sides have the lengths shown. All of the angles in the shape are right angles, as labelled.
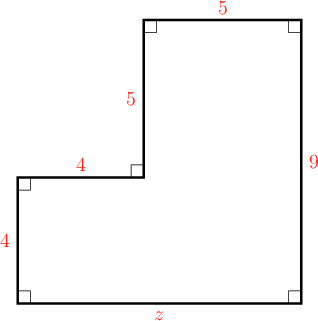
Determine the value of z. Then find the area of this shape. All lengths are in mm.
Start by finding z. All the angles are right angles, so you can use the sides parallel to z, which are 4 mm and 5 mm long.
z=4+5=9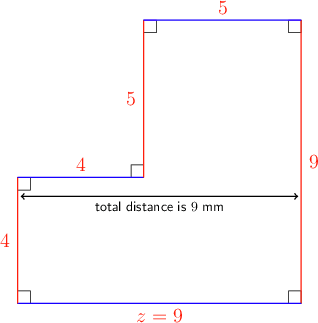
Now we break the shape into two rectangles to find the area.
AreaA=l×b=9×5=45 mm2AreaB=l×b=4×4=16 mm2AreaTotal=AreaA+AreaB=61 mm2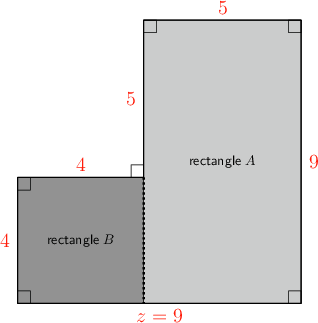
12.4 Practical applications
In real life we often need to calculate a perimeter or area. Then it is useful to know the correct formula as well as how to use the formula.
Worked example 12.24: Finding area in a real life situation
Atinuke is working on a new design to print on fabric. He wants a pink circle with a smaller white circle inside it. The diagram below shows the design.
O is the centre of the two circles. The radius of the small circle is 2 mm, and the radius of the big circle is 6 mm.
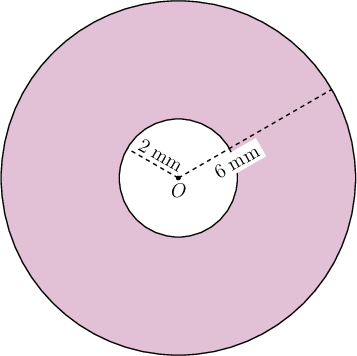
Determine the area of the pink region. Use 3.14 for π.
-
Step 1: Figure out how the design is made.
The design is made from a larger pink circle and a smaller white circle.
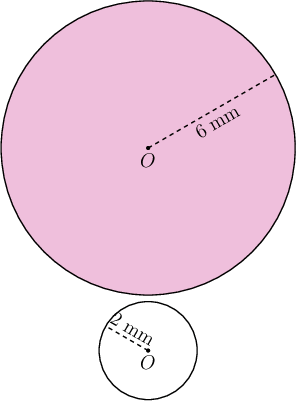
-
Step 2: Make a plan to solve the problem.
To calculate the area that is in pink, we are going to:
- calculate the area of the big pink circle
- calculate the area of the small white circle
- subtract the area of the small circle from the area of big circle.
-
Step 3: Calculate the area of both circles.
When we have two circles of different sizes, we can use R as the variable for the radius of the bigger circle.
Area of big circle:
A1=π×R2=3.14×62=3.14×36=113.04 mm2Area of small circle:
A2=π×r2=3.14×22=3.14×4=12.56 mm2 -
Step 4: Subtract the areas of the circles to get the area of the pink region.
Area of pink region:
A=A1−A2=113.04−12.56=100.48 mm2The area of the pink region is 100.48 mm2.
Exercise 12.12 :Find perimeter and area in real life situations
-
The diagram below shows a notebook with all the measurements given in centimetres.

- Find the perimeter of the notebook.
The notebook is in the shape of a rectangle, so you use the formulae for the perimeter of a rectangle.
P=2l+2b=2×25+2×15=50+ 30=80 cmThe notebook has a perimeter of 80 cm.
-
Find the area of the notebook
Use the formula for the area of a rectangle.
A=l×b=25×15=375 cm2The notebook has an area of 375 cm2.
-
Adanna is renovating her house, and she wants to have a blind made for the window. The blind will be a flat piece of fabric that reaches to the outside edge of the window. The window is in the shape of a square, and at the outside edge, the sides are all 65 centimetres.
- Adanna wants to have a velvet strip around the edge of the blind. Find the perimeter of the window to work out the length of the velvet strip.
The window is in the shape of a square, so use the formula for the perimeter of a square.
P=4s=4×65=260 cmThe perimeter of the window is 260 cm, so the velvet strip will be 260 cm long.
-
Find the area of the window to work out how big the blind has to be.
Use the formula for area of a square.
A=s2=65×65=4,225 cm2The blind will be 4,225 cm2.
-
Talatu paints plates to sell to tourists. She paints the plates purple with a white circle in the centre.
The diagram below shows the plate. O is the centre of the plate. The radius of the small white circle is 3 cm and the radius of the big blue circle is 7 cm.
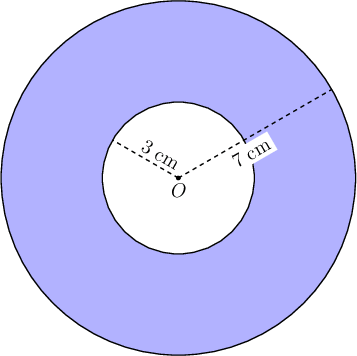
Determine the area of the purple region. Use 3.14 for π.
To calculate the area of the purple region, you need to calculate the area of each of the two circles, and then subtract one area from the other.

Area of big circle:
A1=π×R2=3.14×72=3.14×49=153.86 cm2Area of small circle:
A2=π×r2=3.14×32=3.14×9=28.26 cm2Area of purple region:
A=A1−A2=153.86−28.26=125.6 cm2The area of the purple region is 125.6 cm2.
12.5 Summary
- A two-dimensional (2D) shape has two dimensions that we can measure: we can measure the length and we can measure the breadth (or width) of the shape.
- A polygon is a closed flat shape with three or more straight sides.
- A triangle is a polygon with three sides and three angles.
- A quadrilateral is a polygon with four sides and four angles.
- The following shapes are examples of quadrilaterals: square, rectangle, rhombus, parallelogram, trapezium.
- A circle is a 2D shape with a curved edge, so a circle is not a polygon.
- The perimeter of a circle (the length around the circle) is called its circumference.
- A line segment that goes all the way across the circle and passes through the centre is called a diameter.
- A line segment from the centre of the circle to a point on the circumference is called a radius.
- Perimeter is the distance around the outside of a shape.
- We can use the following formulae to calculate the perimeter of regular polygons.
| Shape | Perimeter formula |
|---|---|
| rectangle | P=2l+2b |
| parallelogram | P=2l+2b |
| square | P=4s |
| rhombus | P=4s |
| triangle | P=side 1+side 2+side 3=s1+s2+s3 |
| trapezium | P=side 1+side 2+side 3+side 4=s1+s2+s3+s4 |
- Area is the amount of flat space taken up by a shape.
- We can use formulae to calculate the area of two-dimensional shapes.
| Shape | Area formula |
|---|---|
| rectangle | A=l×b |
| square | A=s×s=s2 |
| triangle | A=b×h2 |
| parallelogram | A=b×h |
| trapezium | A=h2(a+b) |
| circle | A=π×r2 |