Chapter 5: Addition and subtraction of integers
5.1 Revise place values
In Chapter 1 you learnt that the place value of a digit tells us what the value of that digit is, based on its position in a number. You used place value tables to determine the place value of specific digits in a number. For example, the number two million three hundred and five thousand two hundred and fourteen, may be written in a place value table as follows:
millionhundred thousandten thousandthousandhundredtenunit(1,000,000)(100,000)(10,000)(1,000)(100)(10)(1)2305214The place values of the non-zero digits in 2,305,214 are therefore:
2×1,000,000=2,000,000
3×100,000=300,000
5×1,000=5,000
2×100=200
1×10=10
4×1=4
Exercise 5.1: Determine place values
-
Determine the place value of the digit 8 in each of the following numbers.
- 987
mhthtththhtu(1,000,000)(100,000)(10,000)(1,000)(100)(10)(1)987
The place value is: 8×10=80
- 2,804
mhthtththhtu(1,000,000)(100,000)(10,000)(1,000)(100)(10)(1)2804
The place value is: 8×100=800
- 168,417
mhthtththhtu(1,000,000)(100,000)(10,000)(1,000)(100)(10)(1)168417
The place value is: 8×1,000=8,000
- 3,806,640
mhthtththhtu(1,000,000)(100,000)(10,000)(1,000)(100)(10)(1)3806640
The place value is: 8×100,000=800,000
- 26,008
mhthtththhtu(1,000,000)(100,000)(10,000)(1,000)(100)(10)(1)26008
The place value is: 8×1=8
-
Determine the two place values of the digit 3 in each of the following numbers.
- 363
mhthtththhtu(1,000,000)(100,000)(10,000)(1,000)(100)(10)(1)363
The place values are 300 and 3.
- 3,031
mhthtththhtu(1,000,000)(100,000)(10,000)(1,000)(100)(10)(1)3031
The place values are 3,000 and 30.
- 32,358
mhthtththhtu(1,000,000)(100,000)(10,000)(1,000)(100)(10)(1)32358
The place values are 30,000 and 300.
- 331,047
mhthtththhtu(1,000,000)(100,000)(10,000)(1,000)(100)(10)(1)331047
The place values are 300,000 and 30,000.
- 3,158,325
mhthtththhtu(1,000,000)(100,000)(10,000)(1,000)(100)(10)(1)3158325
The place values are 3,000,000 and 300.
5.2 Addition and subtraction of large numbers
It is important to be aware of place values when we add or subtract large numbers. For example, we cannot subtract hundreds from units, or thousands from tens.
Worked example 5.1: Adding whole numbers
Find the total of 403+628+1,023+49.
-
Step 1: Write the numbers one below the other. Line up the units, tens, hundreds, thousands, and so on.
thhtu403+628+1,023+49 -
Step 2: Add the units column. If the answer is more than 9, write the units of the answer underneath the units column. Write the tens of the answer at the top of the tens column.
3+8+3+9=23 thhtu20403+628+1,023+493 -
Step 3: Add the tens column. Include the tens from Step 2. If the answer is more than 9, write the tens of the answer underneath the tens column. Write the hundreds of the answer at the top of the hundreds column.
2+0+2+2+4=10 thhtu120403+628+1,023+4903 -
Step 4: Repeat Step 3 with the hundreds and thousands. Remember to insert a comma to show thousands.
Hundreds: 1+4+6+0=11 thhtu1120403+628+1,023+49103 Thousands: 1+1=2 thhtu1120403+628+1,023+492,103
Worked example 5.2: Subtracting whole numbers
Subtract 178 from 4,032.
-
Step 1: Write the numbers one below the other. Line up the units, tens, hundreds, thousands, and so on.
thhtu4,032−178 -
Step 2: Subtract the units column. If there are not enough units to subtract from, move one group from the tens column to the units column.
- We cannot subtract 8 from 2.
- There are 3 groups of 10 in the tens column. Move one of them to the units column.
- The units column now has the value 10+2=12.
- The tens column has only 2 groups of 10 left.
- Now we can subtract the units: 12−8=4
-
Step 3: Repeat Step 2 with the tens column. If necessary, move one group from the hundreds column to the tens column.
- We cannot subtract 7 from 2.
- There are no groups in the hundreds column. We must move one group from the thousands column to the hundreds column.
- There are 4 groups of 1,000 in the thousands column. Move one of them to the hundreds column.
- The hundreds column now has 1 group of 1,000. This is equal to 10 groups of 100.
- The thousands column has only 3 groups of 1,000 left.
- Now we can move one group from the hundreds column to the tens column.
- The tens column now has 12 groups of 10.
- The hundreds column only has 9 groups of 100 left.
- Now we can subtract the tens: 12−7=5
-
Step 4: Repeat Step 3 with the hundreds and thousands.
Hundreds: 9−1=8 th0h0t0u391212\cancel4,\cancel0\cancel3\cancel2−178854 Thousands: 3−0=3 th0h0t0u391212\cancel4,\cancel0\cancel3\cancel2−1783,0854
Exercise 5.2: Add and subtract whole numbers
-
Add the following numbers.
- 9,000+800+70+6
9,000+800+70+69,876
- 5,050+55+5+500
1 105,050+55+5+5005,610
- 2,804+1,999+3,333
21102,804+1,999+3,3338,136
- 42+1,005+936+41
111042+1,005+936+412,024
- 909+9,090+9,009+9
1120909+9,090+9,009+919,017
-
Subtract the following numbers.
- 8,642−2,468
513128,\cancel6\cancel4\cancel2−2,4686,174
- 1,723−958
0161113\cancel1,\cancel7\cancel2\cancel3−958765
- 7,777−888
6161617\cancel7,\cancel7\cancel7\cancel7−8886,889
- 10,000−8,734
099910\cancel1\cancel0,\cancel0\cancel0\cancel0−8,7341,266
- 50,000−599
499910\cancel5\cancel0,\cancel0\cancel0\cancel0−59949,401
-
Determine the difference in the two place values of 5 in the following numbers.
To determine the difference between two numbers, subtract the smaller number from the larger number.
- 1,025,568
mhthtththhtu(1,000,000)(100,000)(10,000)(1,000)(100)(10)(1)1025568
The place values are 5,000 and 500.
4001000\cancel5,\cancel000−5004,500- 58,753
mhthtththhtu(1,000,000)(100,000)(10,000)(1,000)(100)(10)(1)58753
The place values are 50,000 and 50.
499100\cancel5\cancel0,\cancel0\cancel00−5049,950- 5,198,352
mhthtththhtu(1,000,000)(100,000)(10,000)(1,000)(100)(10)(1)5198352
The place values are 5,000,000 and 50.
49999100\cancel5,\cancel0\cancel0\cancel0,\cancel0\cancel00−504,999,950
5.3 The number line
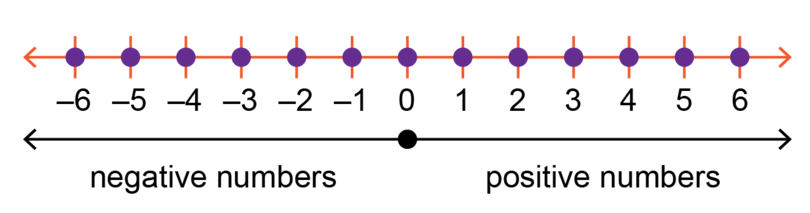
The set of integers is made up of all the positive and negative whole numbers, including zero. We may represent integers on a number line. This is a straight line, divided into equal segments, that shows the position of the numbers.
Numbers to the right of zero are called positive numbers. We represent them with or without a plus sign in front. For example, the positive number five may be written as +5 or just 5. Numbers to the left of zero are called negative numbers. We always represent them with a minus sign in front. For example, the negative number three is written as −3. The number zero is neither positive nor negative.
integers Integers are positive and negative whole numbers, including zero.
number line A number line is a straight line divided into equal segments, that shows the position of numbers.
positive numbers Positive numbers are numbers that are larger than zero. They lie to the right of zero on a number line.
negative numbers Negative numbers are numbers that are smaller than zero. They lie to the left of zero on a number line.
We draw a number line with arrowheads at both ends to show that the line can carry on in both directions forever. Here is an example of a number line:
A number line can also be drawn vertically. Then the positive numbers lie above zero and the negative numbers lie below zero.
Comparing integers on the number line
Positive and negative numbers are sometimes called directed numbers. This is because the sign of the number (positive or negative) tells us in which direction from zero they appear on the number line.
directed numbers Directed numbers are positive and negative numbers. The sign of a directed number tells us in which direction from zero it lies on the number line.
The further to the right a number lies on the number line, the larger it is. The further to the left a number lies on the number line, the smaller it is. We use the symbols < and > to show the relationship between two numbers:
- The symbol < means "smaller than".
- The symbol > means "greater than".
Look at the number line above again. The number 2 lies to the left of the number 6 on the number line. We may write this in two ways:
- 2<6 , which means two is smaller than six
- 6>2, which means six is greater than two
To help you choose the correct symbol, remember that the symbol must "open up" towards the larger number. The "closed" and smaller end points to the smaller number. This crocodile may also help you remember which symbol to use. It always wants to eat the larger number, and it turns away from the smaller number.

Exercise 5.3: Compare integers
-
Draw a number line showing all the integers from −10 to 10.

-
Place the correct symbol between each pair of numbers. Use your number line to help you.
- 1◻7
1<7
- 9◻6
9>6
- 4◻0
4>0
- −4◻0
-4<0
- 1◻−2
1>-2
- −6◻1
-6<1
- −3◻−2
-3<-2
- −3◻−7
-3>-7
- −8◻−5
-8<-5
- 1◻−1
1>-1
-
Arrange the following lists of numbers as required, using the correct symbol. Use your number line to help you.
- Arrange from the smallest to the largest: 1;−5;−3;2;−1
-5<-3<-1<1<2
- Arrange from the largest to the smallest: −9;6;3;−6;0
6>3>0>-6>-9
5.4 Addition and subtraction using the number line
Adding integers
We may use a number line to count. When we count to the right, we count in the positive direction. This is because the numbers become larger as we move to the right on a number line. When we count to the left, we count in the negative direction. This is because the numbers become smaller as we move to the left on a number line.
When we add one integer to another, we may see it as units that are added along the number line. Examples of how we may interpret addition are:
- 5+2: from the number 5, count 2 units in the positive direction (to the right)
- −7+3: from the number −7, count 3 units in the positive direction (to the right)
- 1+(−4): from the number 1, count 4 units in the negative direction (to the left)
- −6+(−3): from the number −6, count 3 units in the negative direction (to the left)
Integers may be added in any order. For example, −1+3 and 3+(−1) give the same answer.
Worked example 5.3: Adding integers using the number line
Use a number line to determine the following: −5+10
-
Step 1: Draw a number line and find the first number on the line.
The first number is −5.
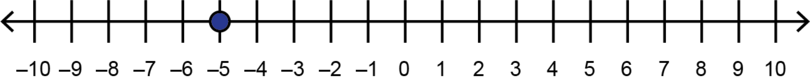
-
Step 2: Determine how many units you must count, and in which direction.
The number 10 indicates that you must count 10 units in the positive direction, that is, to the right.

A positive number does not always have a plus sign in front. When we write 10, it represents +10.
-
Step 3: The answer is the number where you end up.
−5+10=5
Use a number line to determine the following: −4+(−6)
-
Step 1: Draw a number line and find the first number on the line.
The first number is −4.

-
Step 2: Determine how many units you must count, and in which direction.
The number −6 indicates that you must count 6 units in the negative direction, that is, to the left.
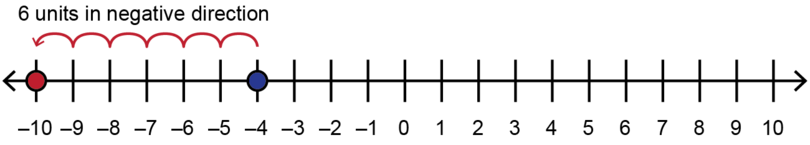
-
Step 3: The answer is the number where you end up.
−4+(−6)=−10
Exercise 5.4: Use a number line to add integers
Use number lines to add the following pairs of integers.
-
1+6
∴1+6=7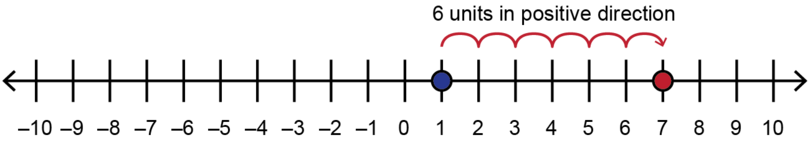
-
−6+7
∴−6+7=1
-
4+(−9)
∴4+(−9)=−5
-
−2+(−6)
∴−2+(−6)=−8
Subtracting integers
There are different ways to think about subtraction. One way is to see it as another form of addition. Subtracting a number is the same as adding the "opposite" of the number. A number and its "opposite" are equal distances from zero, but on opposite sides. For example, −7 is the "opposite" number of 7, and 5 is the "opposite" number of −5.
The "opposite" of a number referred to above has a special name. It is called the additive inverse of the number. When you add a number and its additive inverse, the answer is zero.
The subtraction 6−4 is the same as 6+(−4). Similarly, the subtraction 5−(−1) is the same as 5+(+1). After writing a subtraction as an addition, you may use the method shown in Worked example 5.3 to add the two numbers.
Exercise 5.5: Write subtraction as addition
Write each subtraction as an addition.
-
1−6
-
-
-
Another way is to see subtraction as the difference between two numbers. For example, if you have 7 sweets and you eat 4 sweets, you have left. The number of sweets you have left is the difference between the sweets you had at the start and the sweets you ate. On a number line, the difference between the numbers 7 and 4 is 3 units.
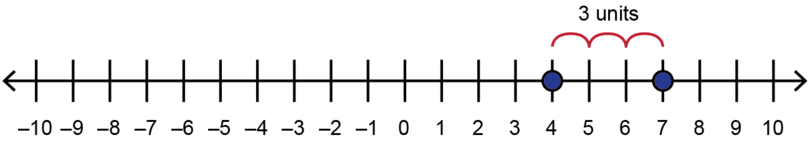
The number 7 lies 3 units to the positive side of the number 4. Therefore, . At the same time, the number 4 lies 3 units to the negative side of the number 7. Therefore, .
Integers cannot be subtracted in any order. For example, and do not give the same answer.
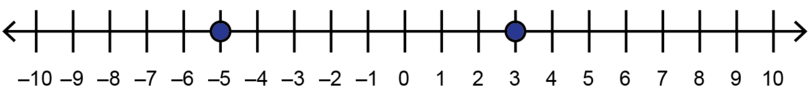
Worked example 5.4: Subtracting integers using the number line
Use a number line to determine the following:
-
Step 1: Identify the two integers that must be subtracted.
The numbers are 5 and 3.
-
Step 2: Draw a number line and show the two numbers on the line.
-
Step 3: Determine the difference between the two numbers.
The difference between 5 and 3 is 8 units.

-
Step 4: Determine whether the number you are subtracting from lies to the positive or the negative side of the number that is being subtracted.
5 lies to the negative side of 3.
-
Step 5: Use the information from Step 4 to decide whether the answer is positive or negative.
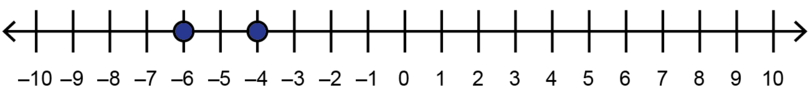
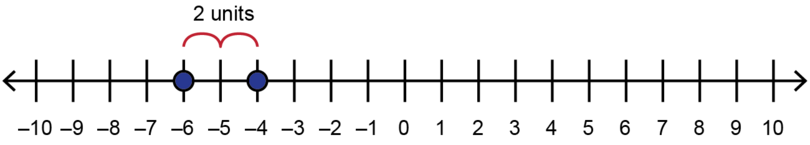
Use a number line to determine the following:
-
Step 1: Identify the two integers that must be subtracted.
The numbers are 4 and 6.
-
Step 2: Draw a number line and show the two numbers on the line.
-
Step 3: Determine the difference between the two numbers.
The difference between 4 and 6 is 2 units.
-
Step 4: Determine whether the number you are subtracting from lies to the positive or the negative side of the number that is being subtracted.
4 lies to the positive side of 6.
-
Step 5: Use the information from Step 4 to decide whether the answer is positive or negative.
Exercise 5.6: Use a number line to subtract integers
For each pair, use a number line to subtract the second integer from the first one.
-

2 lies to the negative side of 5.
-
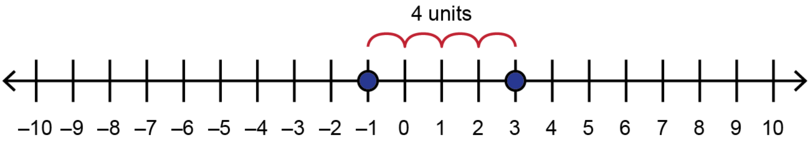
1 lies to the negative side of 3.
-

5 lies to the positive side of 6.
-
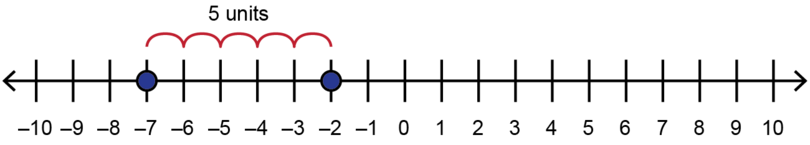
2 lies to the positive side of 7.
Worked example 5.5: Comparing different methods of subtracting integers
Determine the following:
-
Identify the two integers that must be subtracted.
The number 3 must be subtracted from the number 4.
-
Method 1: Regard subtraction as another form of addition.
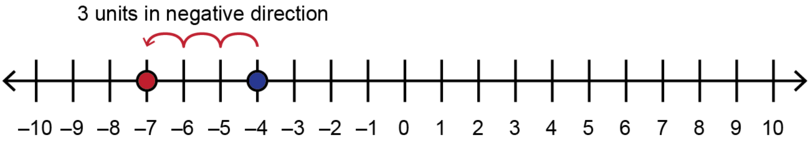
-
Method 2: Regard subtraction as the difference between two numbers.

4 lies to the negative side of 3.
-
The two methods give the same answer.
5.5 Practical applications
Thermometers
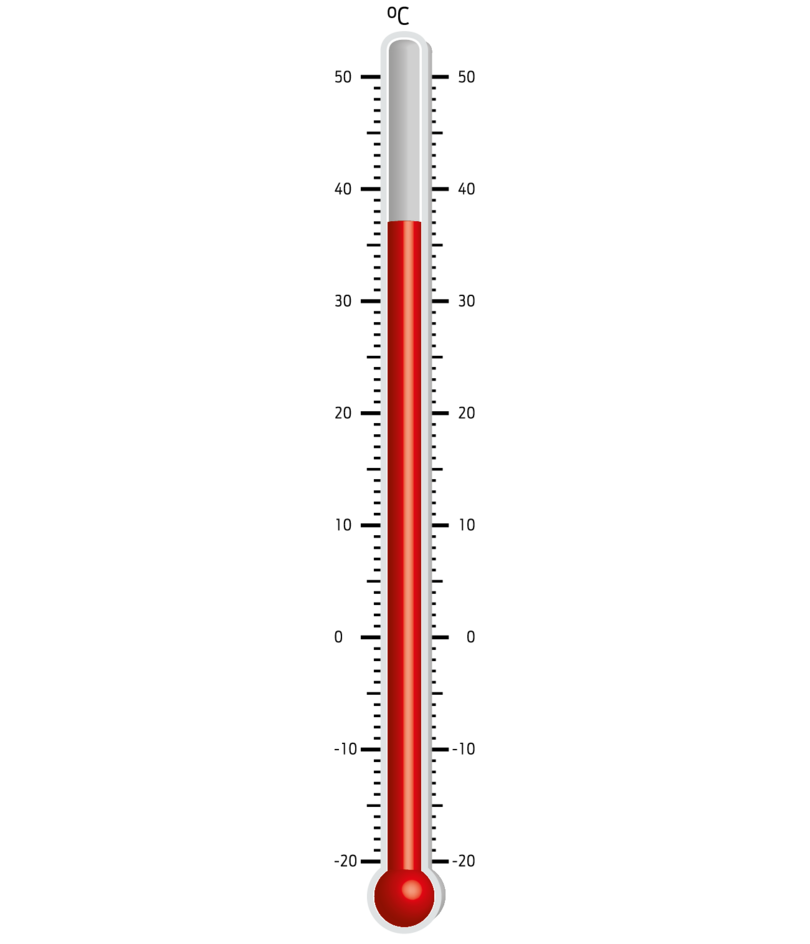
A thermometer is an instrument we use to measure temperature. Most thermometers we use in everyday life measure the temperature in degrees Celsius . The freezing point of water, , is shown somewhere in the middle of the thermometer. Temperatures above are positive and temperatures below are negative. The average body temperature of a healthy human is , as shown on the thermometer below. The typical temperature of the freezing compartment of a refrigerator is .
Banking
Banks allow people to borrow money from them. When you put money into your bank account, you have a positive bank balance. When you draw money from your bank account, it is subtracted from your balance. If you withdraw more money than you have in your bank account, your bank account goes into an overdraft. Your bank balance becomes negative. Part of a bank statement is shown below.
\begin{array}{|l l r|} \hline \ggg \triangleright\triangleright\triangleright & \textbf{BESTBANK NIGERIA}\newline \hline \text{2019/10/01} & \text{Balance brought forward} & ₦120,512.45 \newline \text{2019/10/05} & \text{Deposit} & ₦13,000.00 \newline \text{2019/10/12} & \text{Withdrawal} & -\,₦4,000.00 \newline \text{2019/10/15} & \text{Credit card transaction} & -\,₦52,514.05 \newline \text{2019/10/18} & \text{Refund} & ₦500.00 \newline \text{2019/10/22} & \text{Credit card transaction} & -\,₦7,223.40 \newline \text{2019/10/25} & \text{Withdrawal} & -\,₦80,100.00 \newline \hline \text{2019/10/31} & \text{Balance carried over} & -\,₦9,825.00 \newline \hline \end{array}Movement along the same straight line
When we move along the same straight line, we may use positive and negative numbers to determine our position relative to a starting point. The diagram shows part of a school yard.
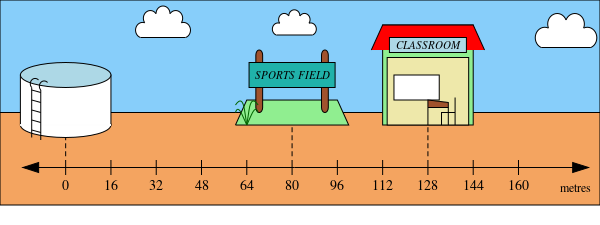
Suppose you walk from the swimming pool 100 m to the right and then 20 m to the left. If distances to the right are taken as positive and distances to the left are taken as negative, you end up to the right of the swimming pool.
Exercise 5.7: Use positive and negative numbers to solve problems.
-
Food is taken out of the freezing compartment of a refrigerator. Its temperature changes from to . Calculate by how much the temperature of the food increased.
To calculate the change in a quantity, use the following principle:
\require{enclose} \begin{array}{r l} 7\,\enclose{circle}[mathcolor="red"]{-}(-10)&=7\,\enclose{circle}[mathcolor="red"]{+}(+10)\newline &=17\space ^\circ \text C \end{array}
The temperature of the food increased by .
-
A piece of meat is at a room temperature of . It is placed in a freezer. The temperature of the meat drops by . Calculate the new temperature of the meat.
If the temperature dropped, we must count 40 units in the negative direction:
Start at the number .
Count 25 units in the negative direction. Now you are at zero.
Count another units in the negative direction. Now you are at .
The new temperature is .
-
Scientists have worked out that the average temperature on the surface of the Earth is , and that the average temperature on the surface of Mars is . Calculate how much warmer than Mars the Earth is.
If we want to know the difference between the temperature of the Earth and of Mars, we must subtract the temperature of Mars:
\require{enclose} \begin{array}{r l} 14\,\enclose{circle}[mathcolor="red"]{-}(-63)&=14\,\enclose{circle}[mathcolor="red"]{+}(+63)\newline &=77\space ^\circ \text C \end{array}The Earth is warmer than Mars.
-
Chioma and Ndidi are sisters. At the start of the school holidays, Chioma owes Ndidi ₦50. Ndidi has another ₦25 that she saved during the term. Both girls earn pocket money during the holidays. They each receive ₦750 in total. Chioma repays her debt to Ndidi. Calculate how much money each of them have at the end of the holidays.
\begin{array}{|l r r|} \hline & \text{Chioma} & \text{Ndidi} \newline \hline \text{Start} & ₦0 & ₦25 \newline \text{Earnings} & ₦750 & ₦750 \newline \text{Debt} & -\,₦50 & ₦50 \newline \hline \text{Balance} & ₦700 & ₦825 \newline \hline \end{array}
-
A man owes the bank ₦5,500. He deposits ₦6,000, but later withdraws ₦800. Calculate his new bank balance.
\begin{array}{|l r|} \hline \text{Start} & -\,₦5,500 \newline \text{Deposit} & ₦6,000 \newline \text{Debt} & -\,₦800 \newline \hline \text{Balance} & -\,₦300 \newline \hline \end{array}
-
Oladapo walks east from his house to school. After walking for 750 m, he realizes that he forgot a book at home. He turns around and walks back. His cell phone rings when he is 200 m from the point where he turned around. Calculate how far from his house Oladapo is when his phone rings.
If east is taken as positive:
He is 550 m to the east of his house.
-
A girl sits in a tree. She throws a ball straight up into the air. It reaches a height of 1 m above the girl's hand and then falls back. The ball falls straight downwards for 3 m, past the tree, and lands on the ground. Calculate the distance between the girl's hand and the ground.
If upwards is taken as positive:
The ground is 2 m below the girl's hand.
-
A bird flies straight north. After 50 m it turns around. It flies straight south for 90 m. It turns around again and flies straight north for 10 m. Determine where it is in relation to its original position.
If north is taken as positive:
The bird is 30 m south of its original position.
5.6 Summary
- The place value of a digit tells us what the value of that digit is, based on its position in a number.
- When we add or subtract large numbers, we line up the units, tens, hundreds and thousands of the numbers.
- A number line is a straight line, divided into equal segments, that shows the positions of numbers.
- Positive numbers are larger than zero and lie to the right of zero on a number line.
- Negative numbers are smaller than zero and lie to the left of zero on a number line.
- Zero is neither positive nor negative.
- The symbol < means "smaller than", and 3<5 means three is smaller than five. The symbol > means "greater than", and 5>3 means five is greater than three.
- When we add one integer to another, we may see it as units that are added along the number line. When we add positive numbers, we count to the right on a number line. When we add negative numbers, we count to the left on a number line.
- Subtracting a number is the same as adding the "opposite" of the number. For example, is the same as .
- Subtraction may also be seen as the difference between two numbers. For example, there are 5 units between the numbers 2 and 7. If the number which is subtracted from lies to the negative side of the number that is subtracted, the difference is negative: . If the number which is subtracted from lies to the positive side of the number that is subtracted, the difference is positive: .
- A thermometer is an instrument used to measure temperature. Temperatures above are positive. Temperatures below are negative.
- A positive bank balance means you have money in the bank. A negative bank balance means you owe the bank money.
- For movement along a straight line, we take one direction as positive. The opposite direction is negative.
